Formula For Binomial Probability

Binomial probability

Table of Contents

Are you struggling to understand the concept of Binomial Probability and its formula? Do you find yourself feeling overwhelmed and intimidated by the math behind it? Fear not, as this article will guide you through the ins and outs of Formula For Binomial Probability, explaining its meaning, uses, and applications.

The Pain Points of Formula For Binomial Probability

For many people, the idea of working with probabilities and distributions can be a daunting prospect. Even people with a strong grasp of math concepts may find themselves struggling when faced with the intricacies of Formula For Binomial Probability. With many different variables and calculations to consider, it’s no wonder that some people find this topic to be confusing and difficult to grasp.

What is Formula For Binomial Probability?

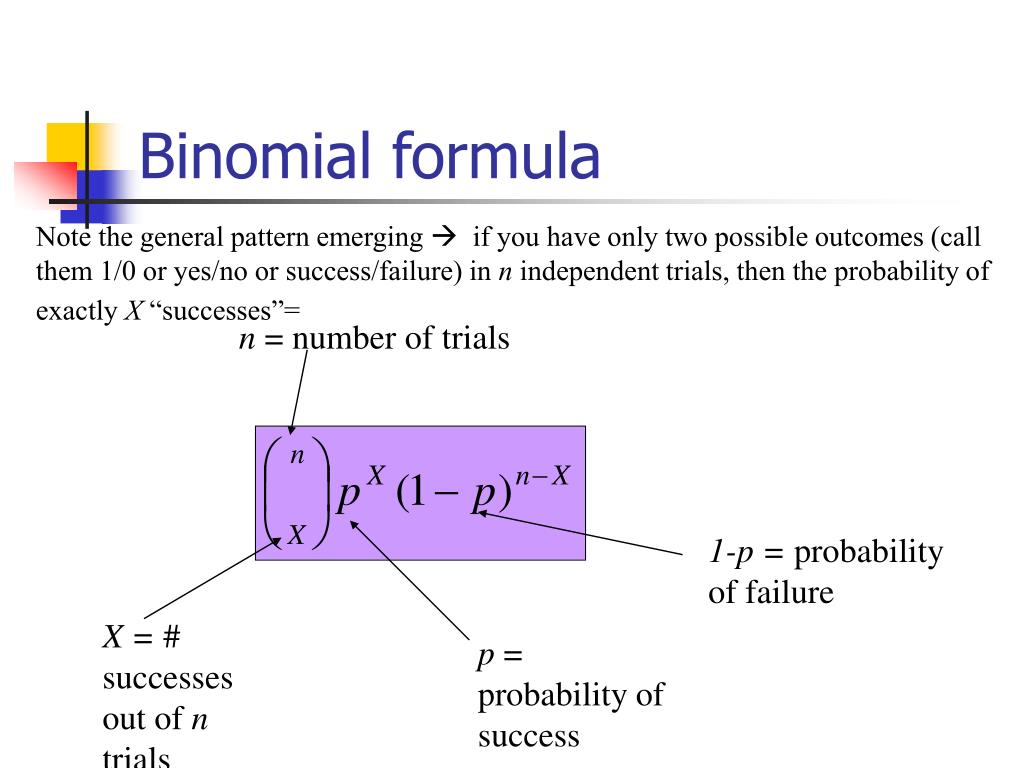
Formula For Binomial Probability is a statistical distribution that describes the likelihood of a particular event occurring a certain number of times in a fixed number of trials. It is used to model situations where there are only two possible outcomes (success or failure), each with a fixed probability, and where each trial is independent of the others. The formula for Binomial Probability is:

This formula can be used to calculate the probability of a range of outcomes, from the most likely to the least likely.

My Personal Experience with Formula For Binomial Probability

As a student, I found Formula For Binomial Probability to be one of the most challenging topics in my math classes. However, with the help of my teacher and some additional resources, I was able to gain a strong understanding of the concept. One of the things that helped me the most was finding real-world examples of how Binomial Probability could be applied in different situations, such as in biology or economics. This helped me to see how powerful the formula could be, and motivated me to continue studying it in more detail.

Applications of Formula For Binomial Probability

Formula For Binomial Probability has many practical applications in fields such as biology, economics, and engineering. It can be used to model a wide range of situations, from coin flips to the outcomes of medical treatments. By understanding the concept of Binomial Probability, you can make more informed decisions and better predict the outcomes of different scenarios.

How to Calculate Formula For Binomial Probability

To calculate Formula For Binomial Probability, you will need to know the number of trials, the probability of success, and the number of successes you are interested in. With these variables in hand, you can plug them into the formula and calculate the probability of getting that number of successes in the given number of trials.

Importance of Understanding Formula For Binomial Probability

Whether you are a student, a researcher, or a professional working in a technical field, understanding Formula For Binomial Probability is essential for making informed decisions and predicting the outcomes of different scenarios. By taking the time to study this topic in detail, you can gain a valuable skillset that will serve you well in a wide range of situations.

How to Improve Your Understanding of Formula For Binomial Probability

If you are struggling to understand Formula For Binomial Probability, there are many resources available to help you. One option is to work with a tutor or mentor who can provide personalized support and guidance. You can also find many online resources, such as video tutorials, interactive simulations, and practice problems, that can help you to reinforce your understanding of this topic.

Question and Answer

Q: What is the difference between Binomial and Poisson Probability?

A: While both Binomial and Poisson Probability are used to model the likelihood of events occurring over time, they are different in several key ways. Binomial Probability is used when there are only two possible outcomes, and the trials are independent of each other. Poisson Probability, on the other hand, is used when the number of successes is proportional to the amount of time or space being measured.

Q: Why is Formula For Binomial Probability important?

A: Formula For Binomial Probability is important because it allows us to make predictions about the likelihood of events occurring, even when we don’t have complete information about the situation. This is particularly useful in fields such as science and economics, where accurate predictions can be crucial for decision-making.

Q: How do you interpret the results of a Binomial Probability calculation?

A: The result of a Binomial Probability calculation is a number between 0 and 1, which represents the probability of getting the specified number of successes in the given number of trials. A result of 0 means that the outcome is impossible, while a result of 1 means that the outcome is certain. Results closer to 0.5 indicate a more unpredictable outcome.

Q: What are some common mistakes to avoid when working with Formula For Binomial Probability?

A: Some common mistakes when working with Formula For Binomial Probability include forgetting to account for all the variables involved (such as the number of trials or the probability of success), misinterpreting the results of calculations, and assuming that the outcomes of one trial will impact the outcomes of subsequent trials.

Conclusion of Formula For Binomial Probability

Formula For Binomial Probability is a powerful tool for predicting the likelihood of events occurring in a wide range of scenarios. Despite its complexity, with a little bit of study and practice, anyone can gain a strong understanding of this important topic.

Gallery

PPT - Binomial Formula, Mean, And Standard Deviation PowerPoint

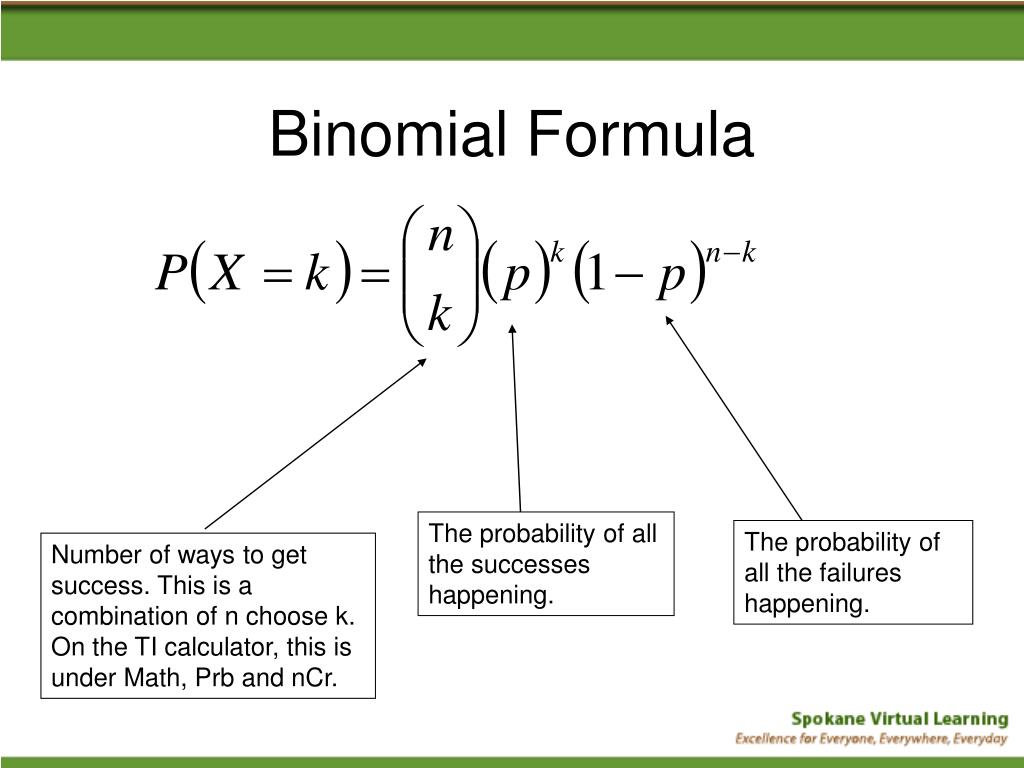
_{^{Photo Credit by: bing.com / binomial formula probability standard deviation success ppt failures successes mean number failure trials then powerpoint presentation}}

PPT - Binomial Probability Distribution 1. The Experiment Must Have A

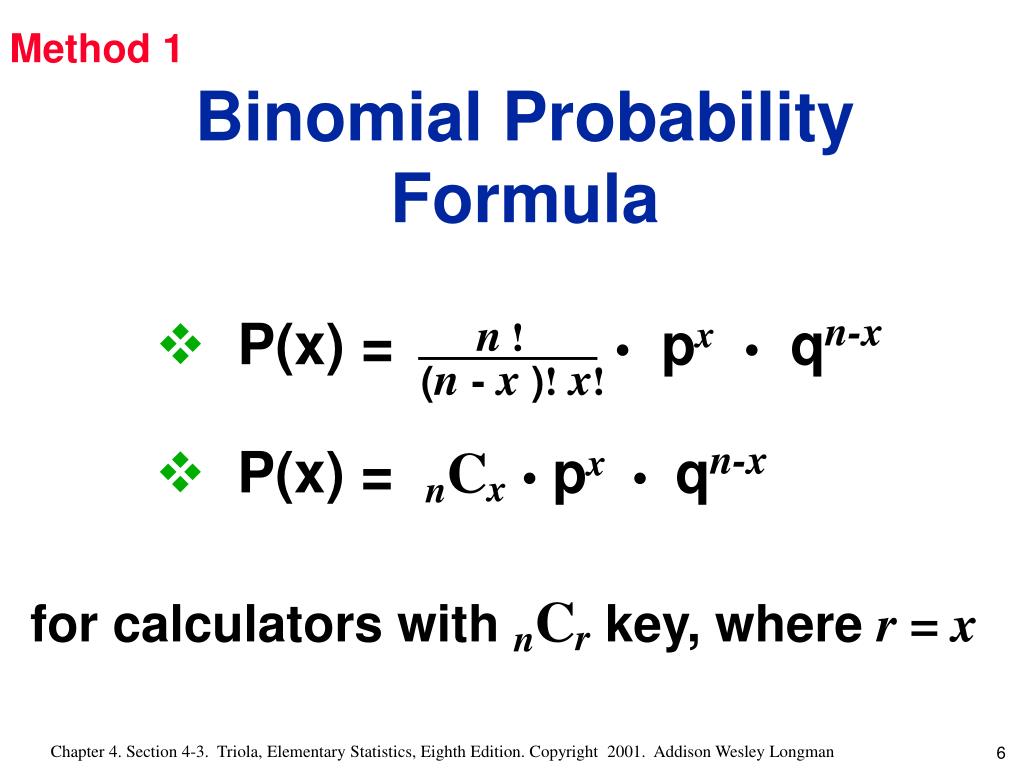
_{^{Photo Credit by: bing.com / binomial probability distribution formula experiment trials fixed number method must ppt powerpoint presentation qn px slideserve}}

Binomial Distribution (examples, Solutions, Formulas, Videos)

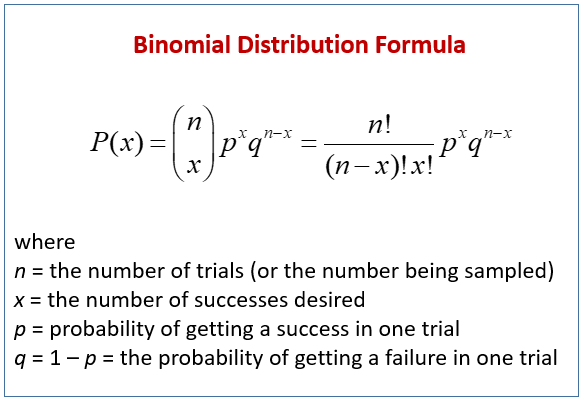
_{^{Photo Credit by: bing.com / binomial distribution formula formulas examples equation solutions distributions stats videos questions introduction}}

PPT - Binomial Probability Formula PowerPoint Presentation - ID:2635610

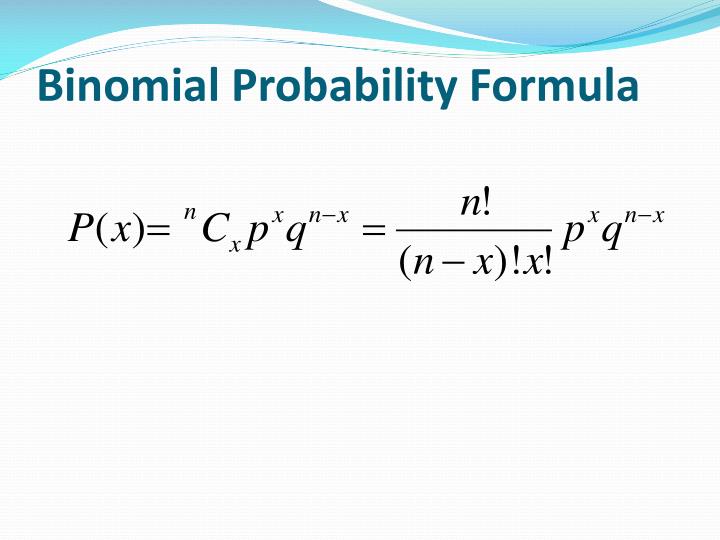
_{^{Photo Credit by: bing.com / binomial probability}}

Binomial Distribution Formula | Calculator (Excel Template)

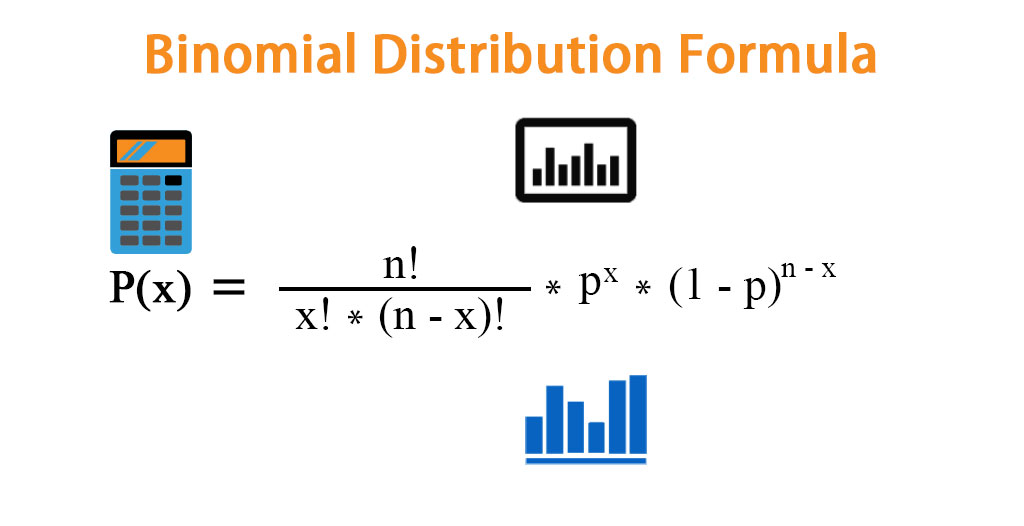
_{^{Photo Credit by: bing.com / binomial probability calculator}}