Binomial Distribution Probability Formula

Binomial distribution examples solutions formulas videos

Table of Contents

Are you curious about Binomial Distribution Probability Formula? This statistical concept can be confusing, but it’s critical to understand for anyone working with data. Keep reading to learn everything you need to know about Binomial Distribution Probability Formula.

The Pain Points of Binomial Distribution Probability Formula

Many people struggle with understanding Binomial Distribution Probability Formula. They may find the formulas and terminology to be intimidating, and may not know how to apply it to real-world situations. Additionally, without a solid grasp of Binomial Distribution Probability Formula, it’s challenging to analyze data and make data-driven decisions.

What is Binomial Distribution Probability Formula?

Binomial Distribution Probability Formula is a statistical concept that shows how many times an event is likely to occur over a set number of trials, assuming a fixed probability for each trial. It’s helpful for analyzing and understanding things like success rates for a given outcome, failure rates, and predicting probabilities in a variety of settings.

Key Points

• Binomial Distribution Probability Formula calculates how often an event is likely to occur over a set number of trials.
• It’s used to analyze success rates for a given outcome, failure rates, and predicting probabilities in a variety of settings.
• Binomial Distribution Probability Formula requires understanding concepts like probability, variance, mean, and standard deviation.

Understanding Key Terms of Binomial Distribution Probability Formula

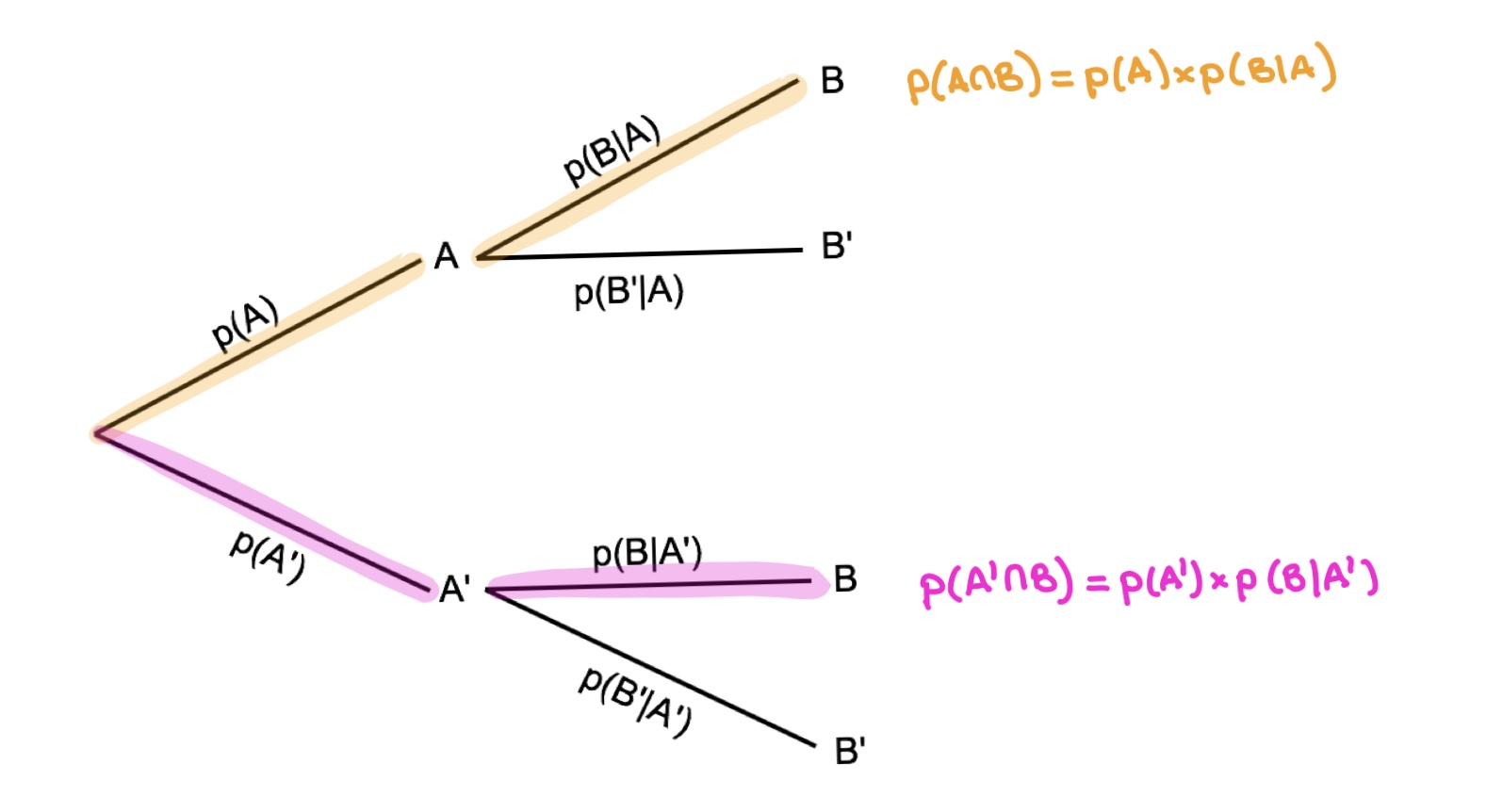
Probability is a measure of how frequently an event is likely to occur. In the context of Binomial Distribution Probability Formula, probability measures the likelihood of success or failure in a given trial. Variance measures how spread out a set of data is, while mean calculates the average of a set of data. Standard deviation measures how much a set of data deviates from the average.

When it comes to Binomial Distribution Probability Formula, understanding these concepts is essential for accurate data analysis and interpretation.

Binomial Distribution Formula Examples:

Let’s say that you’re running an A/B test on your website. You want to test two different headlines on your landing page to see which one results in more clicks. You set up the test to run 100 times and expect a 20% click-through rate (CTR) on each trial.

The Binomial Distribution Probability Formula would allow you to calculate the probability of getting a certain number of clicks with each headline version after 100 trials. This could help you determine which headline is performing better, and make data-driven decisions about your website design.

When to Use Binomial Distribution Probability Formula:

Binomial Distribution Probability Formula is useful in a wide variety of situations. Some common use cases include analyzing survey results, running A/B tests on websites, predicting the outcomes of repeated experiments, and more.

Personal Experience with Binomial Distribution Probability Formula

As a data analyst, I’ve used Binomial Distribution Probability Formula in many different settings. Recently, I used it to analyze the results of a survey we sent out to our customers. By applying the formula, I was able to predict customer behavior and identify areas where we could improve our service offerings. This allowed us to make data-driven decisions and improve customer satisfaction overall.

Binomial Distribution Probability Formula: Calculating the Mean, Variance, and Standard Deviation:

To calculate the mean, variance, and standard deviation for Binomial Distribution Probability Formula, you’ll need to use the following formulas:

• Mean = n * p
• Variance = n * p * (1-p)
• Standard Deviation = sqrt(n * p * (1-p))

Where n is the number of trials, and p is the probability of success in each trial. These formulas are essential for accurate data analysis and should be used whenever working with Binomial Distribution Probability Formula.

Binomial Distribution Probability Formula Applications:

Binomial Distribution Probability Formula has many applications, from predicting customer behavior to analyzing the results of scientific experiments. By understanding this statistical concept, you can make data-driven decisions and improve outcomes for your business or organization.

Question and Answer About Binomial Distribution Probability Formula

Q: What is the difference between Binomial and Poisson distributions?

A: Binomial distributions are used to calculate the probability of a certain number of successes or failures in a set number of trials, assuming a fixed probability of success for each trial. Poisson distributions, on the other hand, are used to calculate the probability of a certain number of events occurring in a fixed interval of time or space, assuming a specific rate of occurrence.

Q: What real-world applications require knowledge of Binomial Distribution Probability Formula?

A: Binomial Distribution Probability Formula is used in many different fields, including finance, marketing, healthcare, social science, and more. It can be applied to analyzing survey results, predicting customer behavior, running A/B tests on websites, and more.

Q: How does Binomial Distribution Probability Formula help businesses?

A: By using Binomial Distribution Probability Formula, businesses can make data-driven decisions that improve outcomes and drive growth. For example, by analyzing the results of an A/B test on a website, businesses can identify design elements that drive higher conversion rates, improving revenue and profitability.

Q: How can I learn more about Binomial Distribution Probability Formula?

A: There are many resources available online that can help you learn more about Binomial Distribution Probability Formula. Resources like online courses, books, and video tutorials can provide valuable insights into this statistical concept and its applications.

Conclusion of Binomial Distribution Probability Formula

Binomial Distribution Probability Formula is a critical concept for anyone working with data. Understanding this statistical concept is essential for accurate data analysis and interpretation, and can help businesses make data-driven decisions that improve outcomes and drive growth. By mastering Binomial Distribution Probability Formula, you can take your data analysis skills to the next level and achieve success in a wide variety of fields.

Gallery

Binomial Distribution (examples, Solutions, Formulas, Videos)

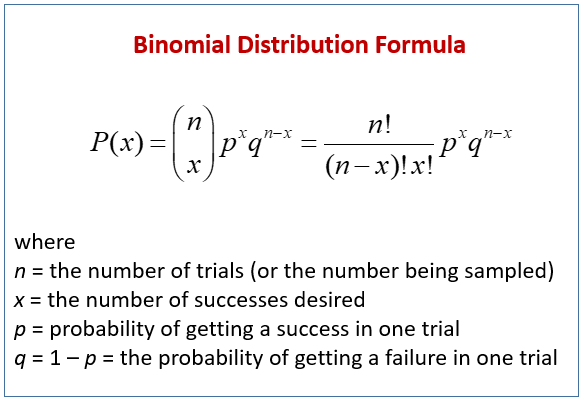
_{^{Photo Credit by: bing.com / binomial distribution formula formulas examples equation solutions distributions stats videos questions introduction}}

Binomial Distribution Formula | Calculator (Excel Template)

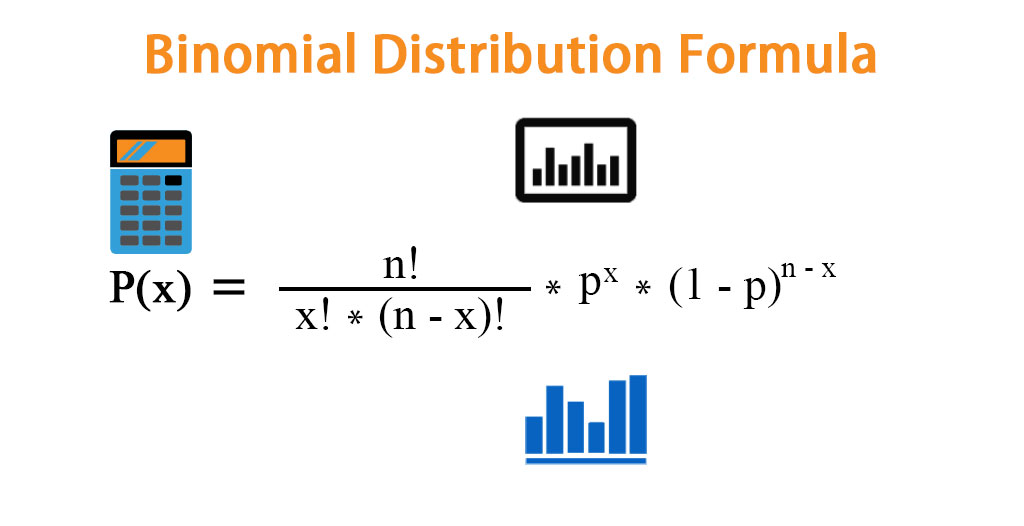
_{^{Photo Credit by: bing.com / binomial probability calculator}}

PPT - Binomial Probability Formula PowerPoint Presentation - ID:2635610

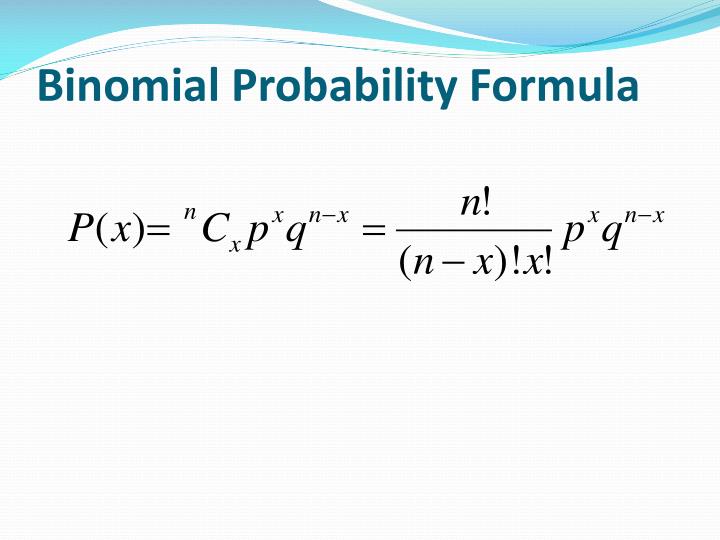
_{^{Photo Credit by: bing.com / binomial probability formula distribution ppt powerpoint presentation}}

PPT - Binomial Probability Distribution 1. The Experiment Must Have A

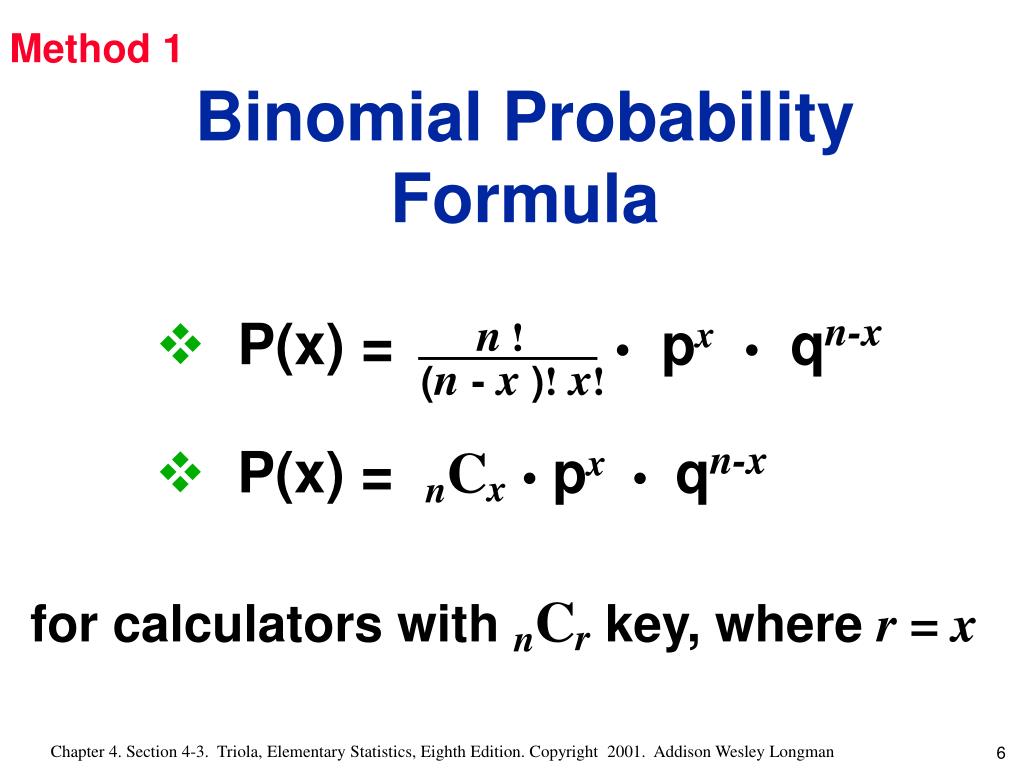
_{^{Photo Credit by: bing.com / binomial probability distribution formula experiment trials fixed number method must ppt powerpoint presentation qn px slideserve}}

PPT - The Binomial Distribution PowerPoint Presentation, Free Download

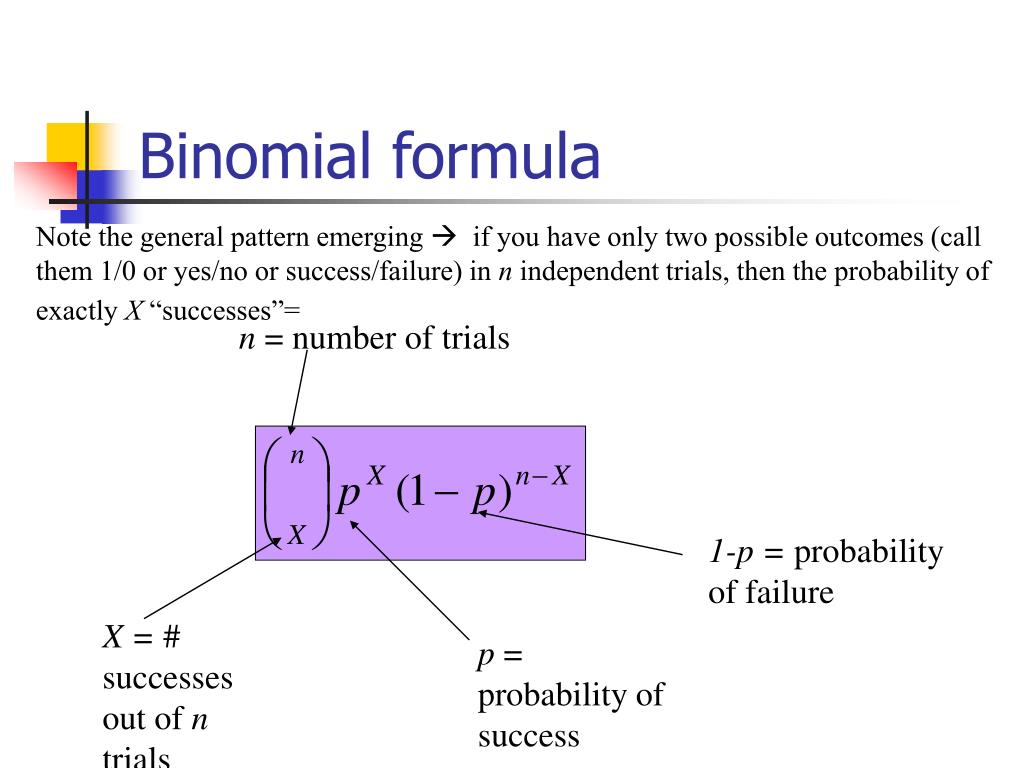
_{^{Photo Credit by: bing.com / binomial distribution formula ppt trials probability general powerpoint presentation number}}