Probability nagwa
Table of Contents
Table of Contents
Are you struggling to understand the concept of Probability Of Two Independent Events? Don’t worry, you’re not alone. Many people find this topic confusing and difficult to grasp. But fear not, by the end of this article, you’ll have a better understanding of Probability Of Two Independent Events and its applications.
Probability Of Two Independent Events can be a complex and daunting topic for many people. Understanding what independent events are and how they relate to each other can be challenging. However, Probability Of Two Independent Events is a crucial concept in probability theory and has many real-world applications in fields such as finance, physics, and engineering.
The target of Probability Of Two Independent Events is to determine the probability of two events occurring independently of each other. This means that the outcome of one event does not affect the probability of the other event occurring. In other words, the probability of both events occurring together is equal to the product of the probabilities of each individual event occurring.
In summary, Probability Of Two Independent Events is the mathematical concept of determining the probability of two events occurring independently of each other. This concept has important applications in many fields, including finance, physics, and engineering.
Understanding Probability Of Two Independent Events
Probability Of Two Independent Events is a crucial concept in probability theory. To understand the concept better, let’s consider a personal experience. Imagine you are playing a game of dice, and you want to know the probability of rolling a six on one die and a two on the other. In this case, since each roll is independent of the other, the probability of rolling a six on one die is 1/6, and the probability of rolling a two on the other die is also 1/6. Therefore, the probability of rolling a six on one die and a two on the other is the product of these two probabilities, which is 1/36.
The Importance of Probability Of Two Independent Events
Probability Of Two Independent Events is an important concept in probability theory and has many real-world applications. For example, it is commonly used in finance to determine the probability of two stocks moving independently of each other. It is also used in physics to determine the probability of two particles interacting independently of each other.
Calculating Probability Of Two Independent Events
Calculating the probability of two independent events is relatively straightforward. To calculate the probability, you simply multiply the probability of each individual event occurring. For example, if the probability of event A occurring is 0.5, and the probability of event B occurring is 0.6, then the probability of both events occurring is 0.3 (0.5 x 0.6).
Real-World Examples of Probability Of Two Independent Events
Probability Of Two Independent Events has many real-world applications. For example, in the stock market, the probability of two stocks moving independently of each other can be calculated using Probability Of Two Independent Events. This can help investors make informed decisions about which stocks to invest in. Similarly, in physics, the probability of two particles interacting independently of each other can be calculated using Probability Of Two Independent Events.
FAQs About Probability Of Two Independent Events
Q: What is the difference between independent and dependent events?
A: Independent events are events where the outcome of one event does not affect the probability of the other event occurring. In contrast, dependent events are events where the outcome of one event affects the probability of the other event occurring.
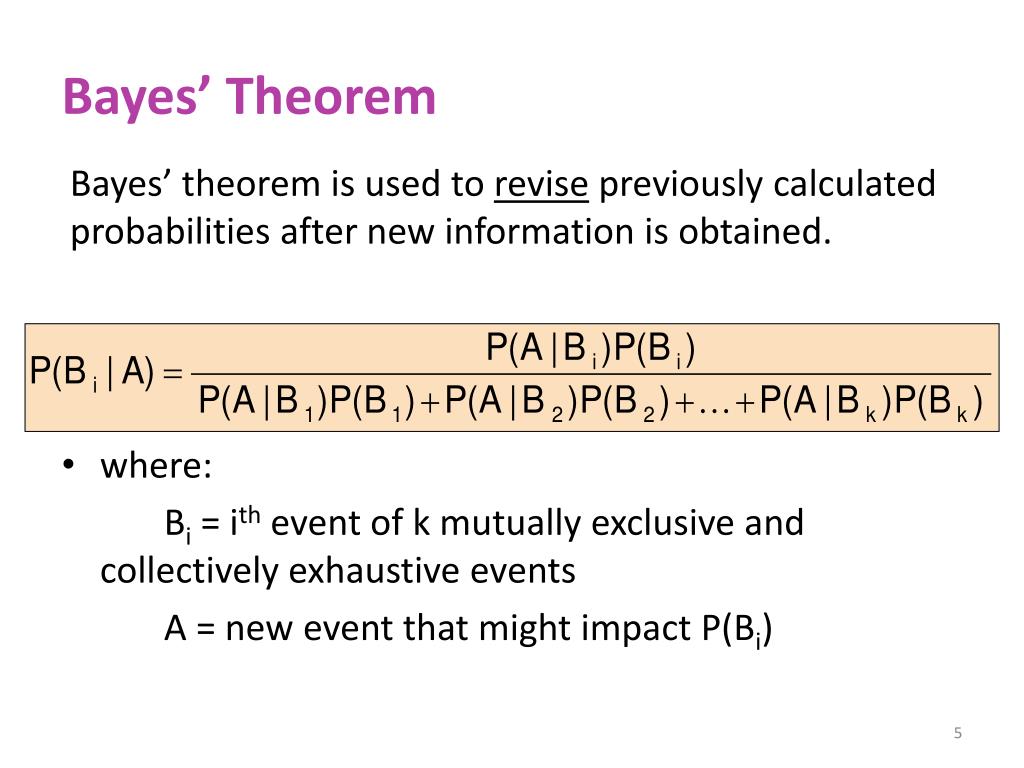
Q: What is the formula for calculating the probability of two independent events?
A: The formula for calculating the probability of two independent events is the product of the probability of each event occurring. That is, P(A and B) = P(A) x P(B).
Q: What are some real-world applications of Probability Of Two Independent Events?
A: Probability Of Two Independent Events has many real-world applications, including finance, physics, and engineering. For example, it is commonly used in finance to determine the probability of two stocks moving independently of each other.
Q: How can I improve my understanding of Probability Of Two Independent Events?
A: One way to improve your understanding of Probability Of Two Independent Events is to practice solving problems involving independent events. You can also seek out online resources, such as videos and tutorials, to help you better understand the concept.
Conclusion of Probability Of Two Independent Events
Probability Of Two Independent Events is a crucial concept in probability theory with many real-world applications. Understanding how to calculate the probability of two independent events and how they relate to each other is essential in fields such as finance, physics, and engineering.
Gallery
Dependent Events (video Lessons, Examples And Solutions)
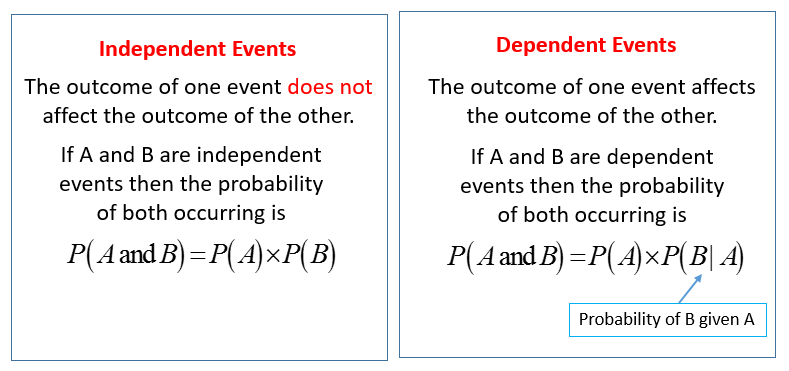
Photo Credit by: bing.com / probability formulas outcome onlinemathlearning
Independent Events (Probability) | Andymath.com
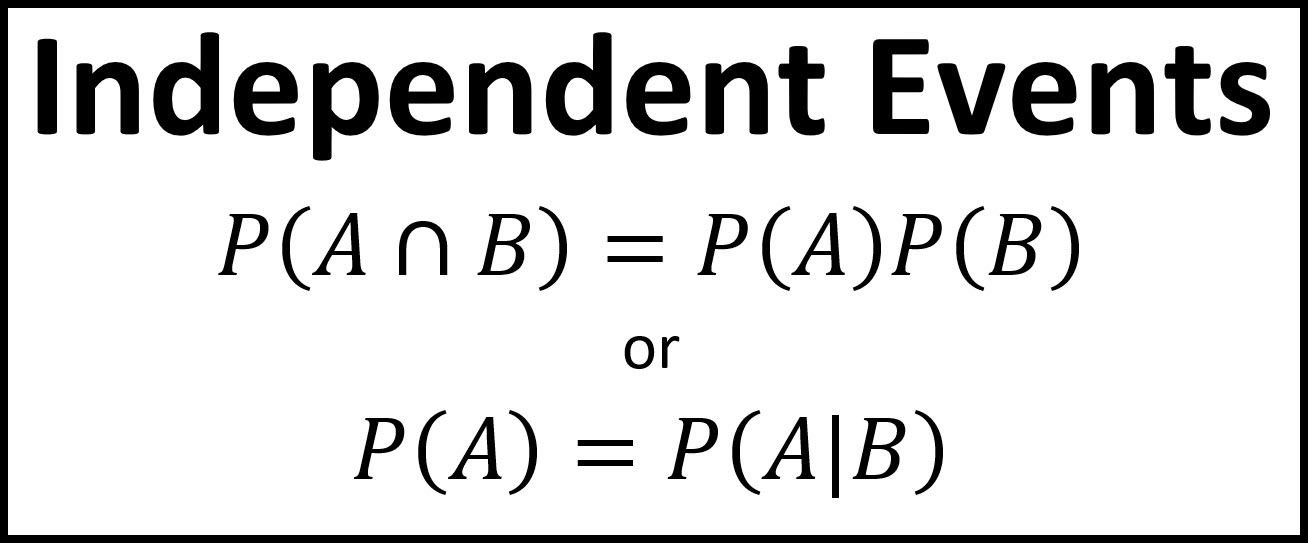
Photo Credit by: bing.com / independent probability events two notes statistics tables way
If A And B Are Two Independent Events, Prove That A’ And B Independent

Photo Credit by: bing.com / independent events if two prove also boards sample class cbse question paper
Question Video: Determining The Probability Of Union Of Two Independent

Photo Credit by: bing.com / probability nagwa
Independent Events (Basics Of Probability: Independence Of Two Events
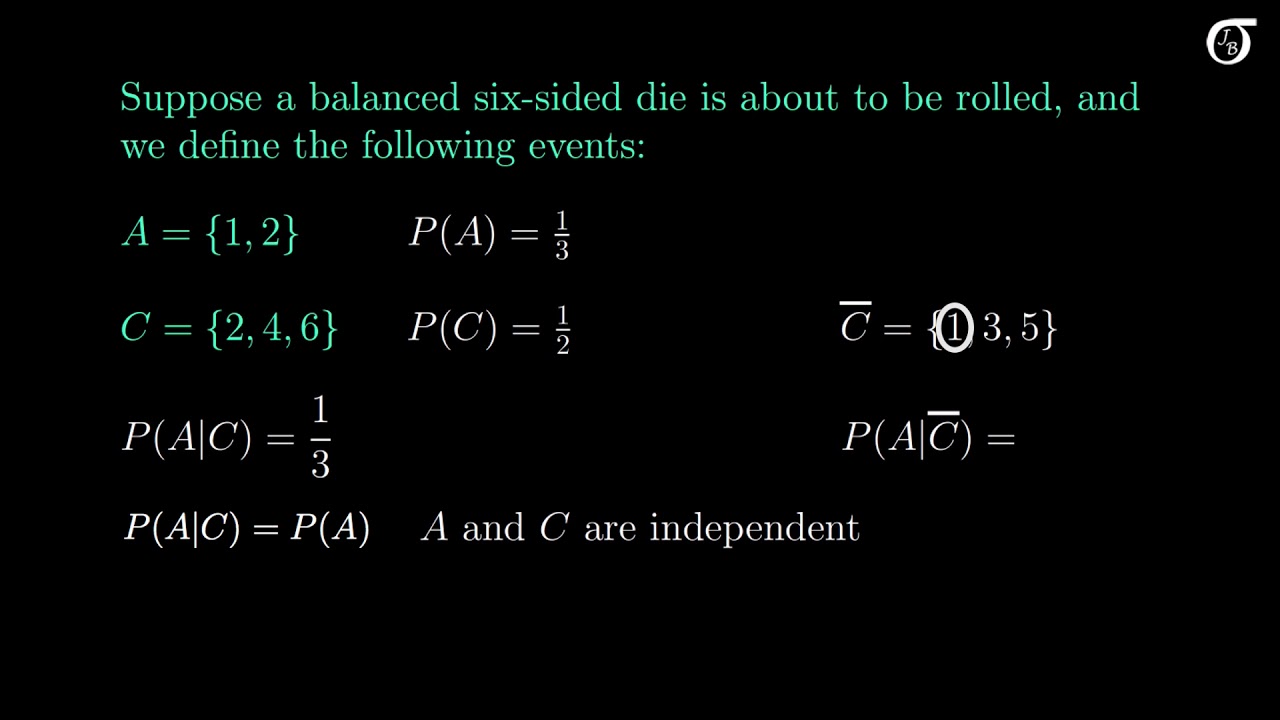
Photo Credit by: bing.com / independent probability events two independence