Independent probability andymath statistics
Table of Contents
Table of Contents
Have you ever wondered about the probability of two independent events occurring? It’s a fascinating topic that can reveal a lot about the likelihood of certain outcomes. In this post, we will dive deep into the world of probability and explore the concept of independent events.
Pain Points
Probability can be a confusing and intimidating topic for many people. It involves complex formulas and calculations, and the idea of two independent events occurring at the same time can seem almost impossible. Many people struggle with understanding the concept of independent events and how they can impact the outcome of a situation.
Target of Probability of 2 Independent Events
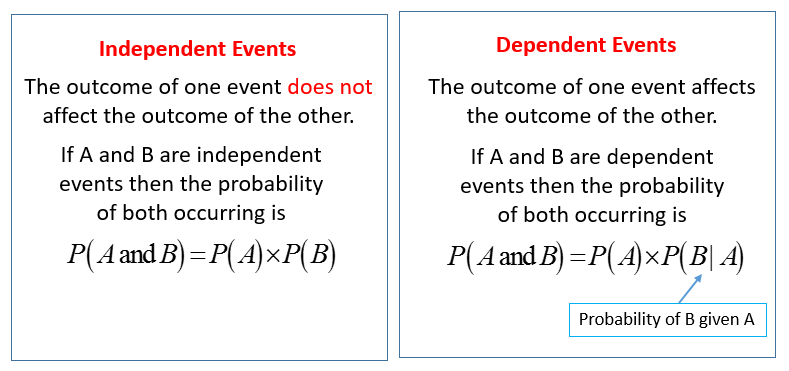
The target of probability of two independent events is to calculate the likelihood of two events occurring at the same time. Two events are considered independent if the outcome of one does not affect the outcome of the other. For example, flipping a coin and rolling a die are two independent events.
Summary
In summary, understanding the probability of two independent events can give us insights into the likelihood of certain outcomes. While it can be a complex topic, breaking down the formulas and understanding the concept of independent events can make it easier to understand.
The Target of Probability of 2 Independent Events
The target of probability of two independent events is to calculate the likelihood of two events occurring at the same time, independent of each other. For example, flipping a coin and rolling a die are two independent events. The probability of getting heads on a coin and rolling a six on the die is the same as the probability of getting heads on the coin and rolling any other number on the die. This is because the outcome of one event (the coin flip) does not affect the outcome of the other event (rolling the die).
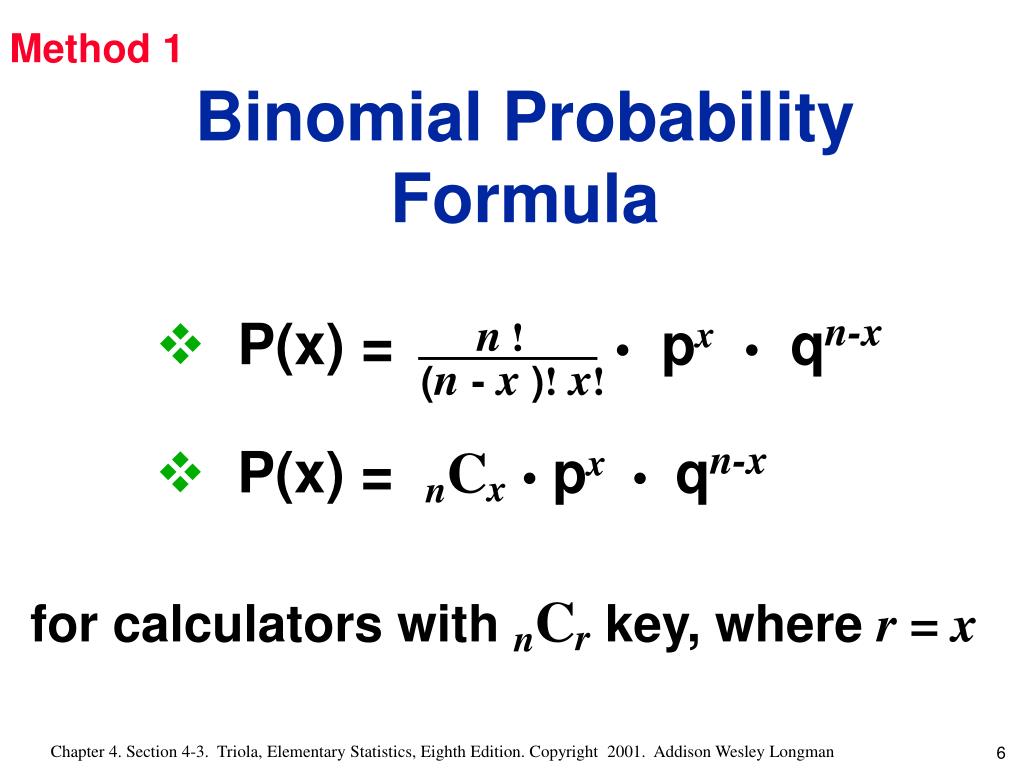
When calculating the probability of two independent events occurring, we use a formula. The formula is as follows:
In this formula, P(A) represents the probability of event A occurring, and P(B) represents the probability of event B occurring. To find the probability of both events occurring at the same time, we multiply these two probabilities together.
Personal Experience
When I was in college, I took a class on probability theory. At first, I found the topic overwhelming and confusing. However, as I spent more time studying and practicing the formulas, I began to understand the concepts more clearly. It was fascinating to see how the probability of two independent events could impact the likelihood of certain outcomes. I especially enjoyed working with real-life examples and seeing how probability theory could be applied in many different fields.
Probability of Independent Events in Real Life
The concept of independent events is used in many different areas of life. For example, the insurance industry uses probability theory to determine the likelihood of certain events occurring, such as car accidents or natural disasters. Stock traders also use probability theory to make investment decisions based on the likelihood of certain stock prices rising or falling.
The concept of independent events can also be applied to everyday situations. For example, if you are planning a weekend trip and need to book a hotel and a rental car, the probability of getting a good deal on both may be independent of each other. The outcome of booking a hotel does not affect the outcome of booking a rental car, so the events are considered independent.
Exploring Probability of 2 Independent Events in More Detail
While the formula for calculating the probability of two independent events is relatively straightforward, there are some important concepts that are worth exploring in more detail. For example, the concept of mutually exclusive events can impact how we calculate probability.
Two events are considered mutually exclusive if the occurrence of one event precludes the occurrence of the other. For example, if you are flipping a coin, the events of getting heads and getting tails are mutually exclusive, because you cannot get both at the same time.
Questions and Answers about Probability of 2 Independent Events
1. What is the formula for calculating the probability of two independent events?
A: The formula is P(A) x P(B), where P(A) represents the probability of event A occurring, and P(B) represents the probability of event B occurring.
2. How do you know if two events are independent?
A: Two events are considered independent if the outcome of one event does not affect the outcome of the other event.
3. What is the difference between independent and mutually exclusive events?
A: Independent events are events where the outcome of one event does not affect the outcome of the other event. Mutually exclusive events are events where the occurrence of one event precludes the occurrence of the other event.
4. Can you give an example of two independent events?
A: Flipping a coin and rolling a die are two independent events, because the outcome of one does not affect the outcome of the other.
Conclusion of Probability of 2 Independent Events
The concept of probability of 2 independent events can be a challenging topic to understand, but it can also provide valuable insights into the likelihood of certain outcomes. By breaking down the formulas and understanding the concepts behind independent events, we can gain a deeper understanding of the world around us.
Gallery
Independent Events Formula In Probability
Photo Credit by: bing.com / probability examples wisenet samsung smartcam sna
Probability And Random Variable Lecture(3)
Photo Credit by: bing.com / probability
Dependent And Independent Events Probability Algebra 2 Regents - YouTube
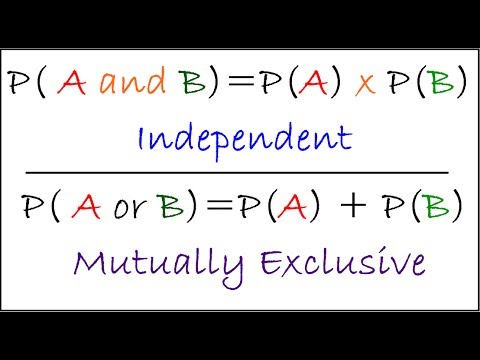
Photo Credit by: bing.com / probability independent events dependent algebra
Independent Events (Probability)
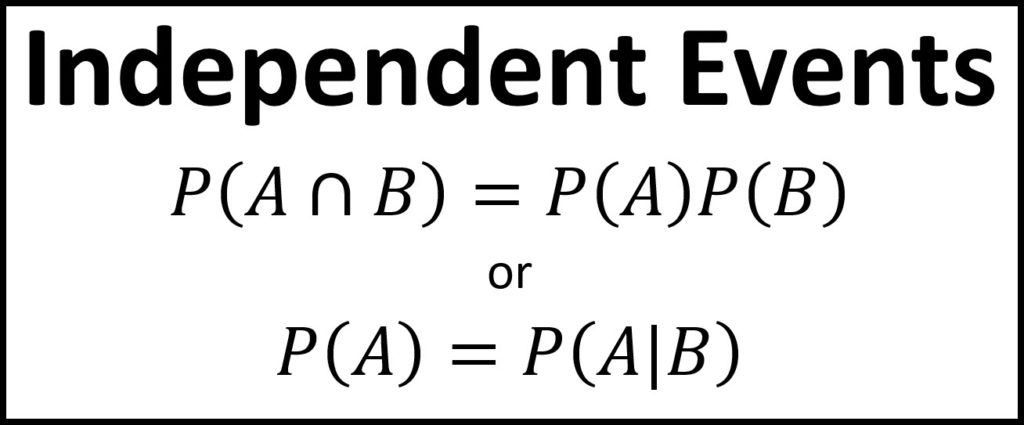
Photo Credit by: bing.com / independent probability andymath statistics
Dependent Events (solutions, Examples, Videos)
Photo Credit by: bing.com / probability formulas outcome
