Difference Between Empirical And Theoretical Probability

Empirical probability

Table of Contents

Have you ever wondered about the chances of winning the lottery or the probability of flipping heads on a coin toss? Probability is all around us, and understanding the difference between empirical and theoretical probability can greatly impact our decision-making.

The Pain Points of Understanding Empirical and Theoretical Probability

Many people struggle to differentiate between empirical and theoretical probability. This can lead to confusion when making important decisions that involve probability, such as investing in the stock market or predicting the outcome of a sports game. Without a proper understanding of these two types of probability, individuals may rely on irrational thinking or biased opinions, leading to suboptimal results.

Understanding the Target of Empirical and Theoretical Probability

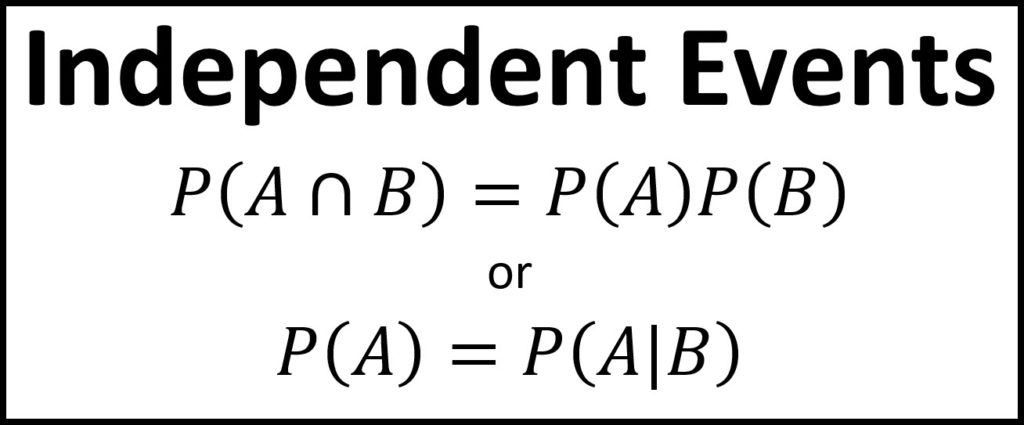
Empirical probability refers to the chance of an event occurring based on past experience or experimental data. On the other hand, theoretical probability is calculated using mathematical formulas and assumptions. While empirical probability is based on real-world observations, theoretical probability is based on theoretical models and assumptions.

Summary of Main Points

Understanding the difference between empirical and theoretical probability is crucial for making informed decisions. Empirical probability is based on past experience, while theoretical probability is based on mathematical models and assumptions. Without a proper understanding of these types of probability, individuals may make suboptimal choices in important situations.

The Target of Empirical and Theoretical Probability

When I was in high school, I took a statistics class that introduced me to the concept of probability. I remember being fascinated by the idea that I could use probability to predict the outcome of a coin toss or the chances of drawing a certain card from a deck. However, it wasn’t until I learned about the difference between empirical and theoretical probability that I truly grasped the concept.

Empirical probability is often used in scientific experiments and research studies. For example, a biologist may use past data to determine the chance of a certain species surviving in a particular environment. In contrast, theoretical probability is often used in mathematics and statistics, such as calculating the probabilities of different outcomes in a game of poker.

The Benefits of Understanding Empirical and Theoretical Probability

Understanding the difference between empirical and theoretical probability can greatly benefit individuals in various fields. For example, investors can use empirical probability to make informed decisions about which stocks to invest in, while scientists can use theoretical probability to make predictions about the behavior of particles in a chemical reaction.

A Closer Look at Empirical and Theoretical Probability

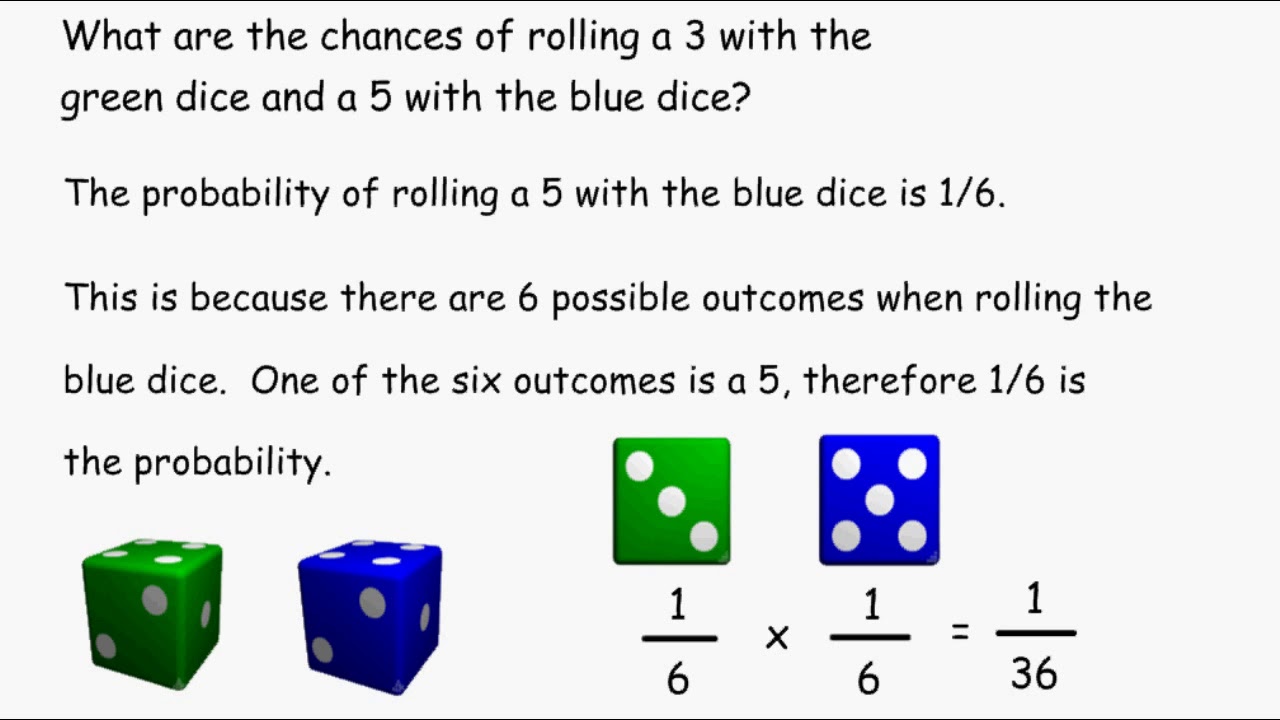
Empirical probability is calculated by dividing the number of times an event occurs by the total number of trials or observations. For example, if a coin is flipped 100 times and lands on heads 50 times, the empirical probability of getting heads would be 0.5 or 50%. On the other hand, theoretical probability is based on mathematical formulas and assumptions. For example, the theoretical probability of rolling a six on a standard die is 1/6, or approximately 0.17.

Real-World Applications of Empirical and Theoretical Probability

Empirical and theoretical probability have many real-world applications. For example, businesses can use empirical probability to predict consumer behavior and make informed marketing decisions. Theoretical probability can be used to predict the outcome of sports games or weather patterns, helping individuals make informed decisions based on data and mathematical models.

Question and Answer

What is the difference between empirical and theoretical probability?

Empirical probability is based on past experience or experimental data, while theoretical probability is based on mathematical formulas and assumptions.

How is empirical probability calculated?

Empirical probability is calculated by dividing the number of times an event occurs by the total number of trials or observations.

What is an example of theoretical probability?

An example of theoretical probability is the probability of rolling a specific number on a die based on mathematical formulas and assumptions.

Why is understanding the difference between empirical and theoretical probability important?

Understanding the difference between empirical and theoretical probability is important for making informed decisions in various fields, such as investing, science, and sports. Without a proper understanding of these types of probability, individuals may rely on irrational thinking or biased opinions, leading to suboptimal results.

Conclusion of Difference Between Empirical And Theoretical Probability

Probability is a powerful tool that can be used in various fields, but understanding the difference between empirical and theoretical probability is crucial for making informed decisions. Empirical probability is based on past experience, while theoretical probability is based on mathematical models and assumptions. By understanding these types of probability, individuals can make informed decisions in important situations, leading to better outcomes.

Gallery

20 Best Images About Probability On Pinterest | Trees, A Tree And Student

_{^{Photo Credit by: bing.com / probability experimental theoretical vs grade math}}

Empirical Probability | Experiment Of Rolling A Die| Tossing Two Coins

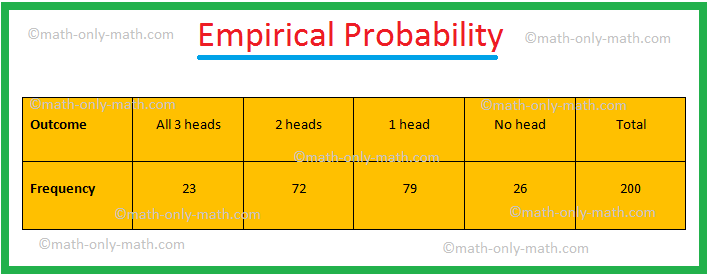
_{^{Photo Credit by: bing.com / probability empirical frequencies tossed simultaneously tossing}}

Theoretical Probability And Experimental Probability (solutions

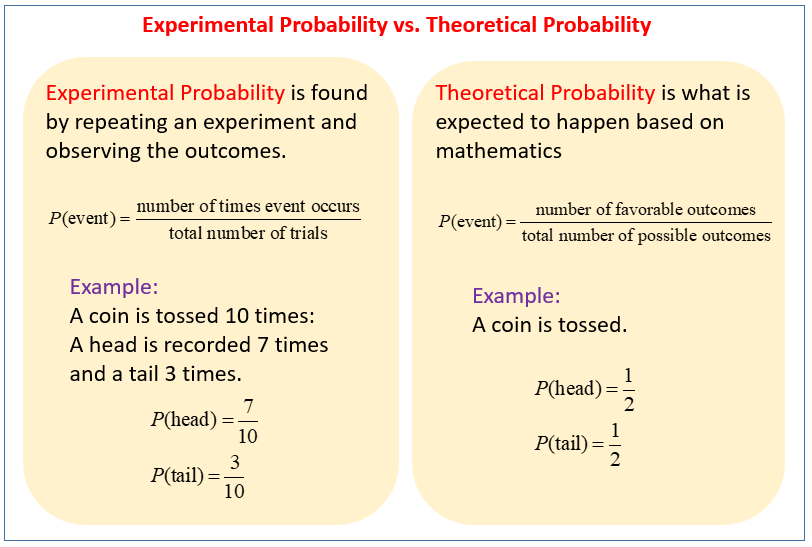
_{^{Photo Credit by: bing.com / probability theoretical experimental solutions}}

What Is The Difference Between Theoretical And Empirical - Slidesharetrick

_{^{Photo Credit by: bing.com / empirical theoretical slidesharetrick pediaa summary}}

What Are The Definitions Of Classical Probability, Empirical

_{^{Photo Credit by: bing.com / probability empirical subjective classical definitions}}