Normal distribution curve life examples real standard deviation mean graph under width height determines center
Table of Contents
Table of Contents
A fascinating aspect of statistics is the definition of normal probability curve, also known as the Gaussian bell curve. This curve is ubiquitous in our world, and understanding its definition unlocks a wealth of knowledge in numerous fields. From finance to healthcare, the normal probability curve plays a pivotal role in decision-making processes. So, what exactly is the definition of normal probability curve, and how does it work?
Many people struggling in their field often feel intimidated by statistics. They believe that analyzing and interpreting data is a task only accomplished by the most informed and intelligent people on the planet. However, normal probability curve is not that difficult to comprehend. Once you understand its concept, understanding it’s application becomes much easier. Therefore, one of the primary pain points associated with a definition of normal probability curve is self-doubt.
In simple terms, the normal probability curve is a continuous probability distribution that lays the foundation of various information about the target parameter like mean and standard deviation. This curve can occur in natural or human-made phenomena. Statistical experts apply normal probability curves in various areas to make informed predictions, control data variation, and improve quality measurements in healthcare, economics, finance, and other fields.
The main points related to the definition of normal probability curve include: understanding the concept of continuous probability distribution, being able to explain it in simple terms, and understanding its real-world applications. The curve follows a specific formula based on the mean and standard deviation of a dataset, and it has a symmetrical bell shape where the data is grouped around the mean.
What Is Normal Probability Curve?
Normal probability curve is a representation of how data is distributed in a specific dataset. The curve is symmetrical and bell-shaped around the mean, with the data concentrated in the middle and gradually decreasing as it moves away from the center. Its primary purpose is to describe and compare data sets in various fields, from social sciences to the natural sciences.
Looking back at my high school years, I recall hearing about normal probability curve in math class, specifically during statistics lessons. I struggled to comprehend it initially, but I remember my teacher showing different shapes of the curve that helped me understand its concept. One critical factor to remember is that the curve remains the same despite the different parameters’ values.
Applications of Normal Probability Curve
The definition of normal probability curve finds applications in many areas. As previously mentioned, finance, healthcare, and economics are a few of the areas that use the curve regularly. One area where it’s application is critical in healthcare is to help identify abnormal test results. For example, identifying these abnormal results can assist in identifying the diagnosis and move forward with the correct treatment.
In finance, the curve can assist in various applications like evaluating stock investment strategies that stabilize and maximize returns. In economics, it plays a significant role in central banking policies that aim to stabilize market fluctuations.
Use of Z-score
Z-score is a critical factor in understanding the normal probability curve. Essentially, it helps to standardize the data points in datasets, making it easier to compare and draw inferences. Z-score is the number of standard deviations away from the mean. This score helps to determine how far or close data samples are from the mean value. Often used in market analysis and financial modeling. It’s a standard calculation applied alongside the normal probability curve to measure the severity of fluctuations and deviations.
Real-Life Example of Normal Probability Curve
One of the best examples of the normal probability curve in action is the analysis of student exam scores. Educational institutions continuously analyze student data to evaluate academic progress, identify areas of improvement, measure teacher performance, and adjust curriculum. Data analysts use the normal probability curve methodology to determine the distribution of exam scores, identify average test scores, and distinguish the most significant and least significant score variations. Not only do they look at specific subjects as the curve can also monitor the overall school’s performance.
Question and Answer
Q: What is the normal distribution curve?
A: It’s a probability distribution that describes the statistical distribution of variables.
Q: Why is the normal distribution curve important?
A: The normal probability curve is essential because it provides valuable insight into how data is distributed, making it a valuable tool in many fields, from healthcare to finance.
Q: What does the area under the normal distribution curve represent?
A: The area below the normal distribution curve represents the total probability of all events occurring in that distribution. The sum of all areas under the normal curve adds up to one (100%).
Q: What are the characteristics of normal distribution curve?
A: The normal distribution curve has a symmetrical shape with an ideal bell-shaped curve, with the mean, the median, and the mode all being the same value.
Conclusion of Normal Probability Curve
Understanding the definition of normal probability curve opens up a world of data analysis potential. Despite the concept appearing challenging initially, it is relatively straightforward once you engage in it. Applying it can help bring insights to various fields’ data, identify patterns, and provide valuable insights. I hope this post has helped you better understand the concept and its potential applications.
Gallery
The Normal Distribution Table Definition

Photo Credit by: bing.com / probability investopedia explained sabrina jiang
The Normal Distribution Table Definition

Photo Credit by: bing.com / probability investopedia explained jiang
The Standard Normal Distribution | Examples, Explanations, Uses
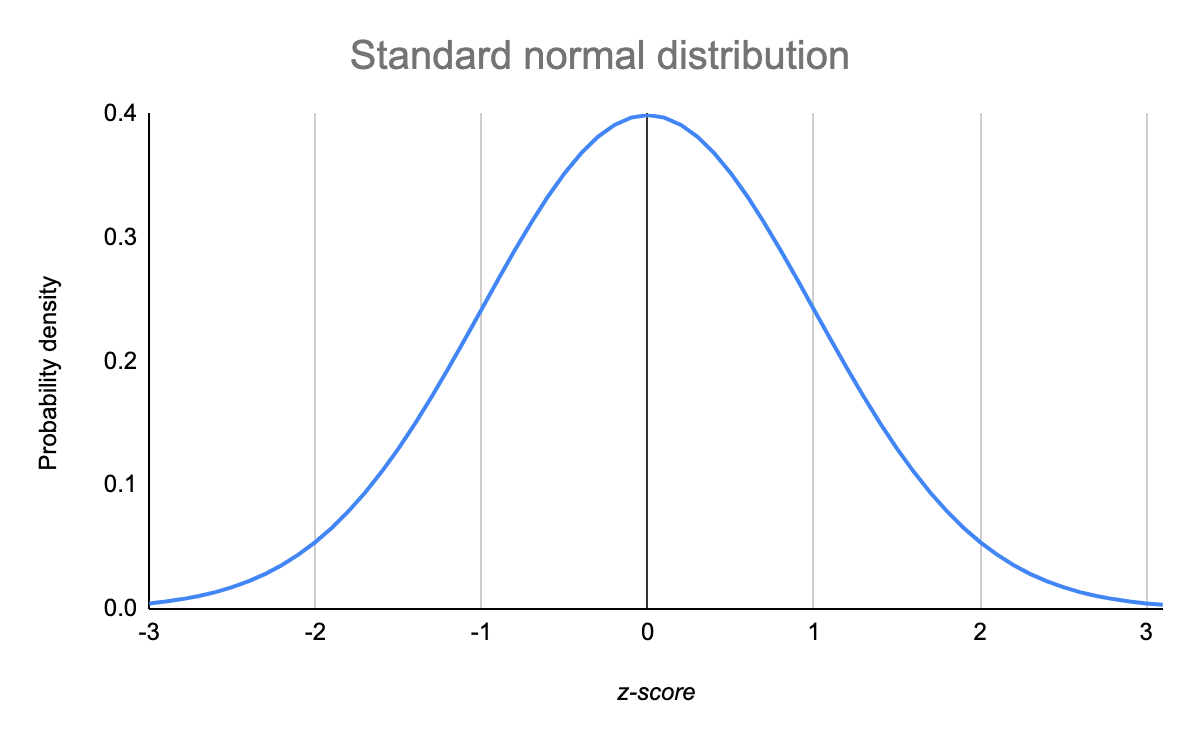
Photo Credit by: bing.com / probability distributions deviations values statistics standardized latency percentile courses fps bimodal spending converting
The Scores On Standardized Admissions Test Are Normally Distributed
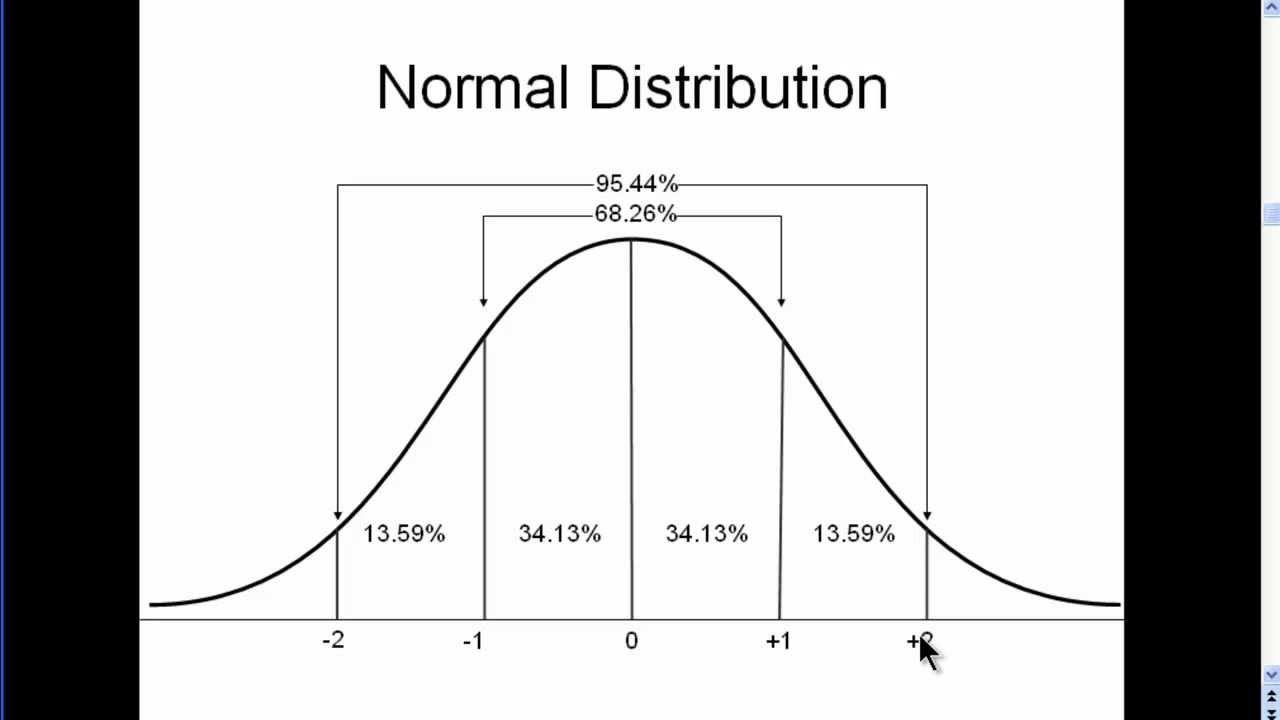
Photo Credit by: bing.com / distribution normal curve mean bell standard deviation statistics normally distributed explained probability make between scores relationship look simply standardized shifted
9 Real Life Examples Of Normal Distribution – StudiousGuy
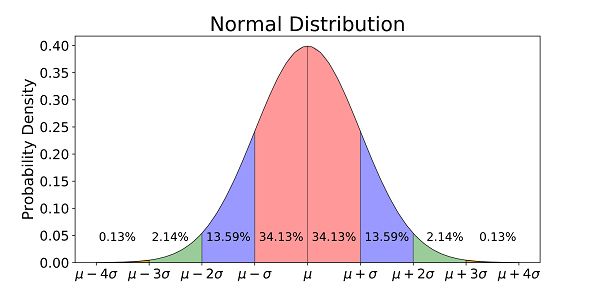
Photo Credit by: bing.com / normal distribution curve life examples real standard deviation mean graph under width height determines center