Define Binomial Probability Distribution

Probability by binomial distribution solved numerical problems

Table of Contents

The world is surrounded by probabilities and statistics. In all our daily activities, there are chances of an event happening or not happening. The probabilities of such events are called Probability Distribution, and one of them is the Binomial Probability Distribution. In this blog post, we will define Binomial Probability Distribution, its target, and explain related keywords.

When making decisions that require high precision, it’s important to understand the probability of the outcome. The Binomial Probability Distribution is one of the essential tools in statistics that helps in understanding and calculating probabilities when we have a set of parameters that falls into either of two outcomes, such as Yes/No or Pass/Fail.

The Binomial Probability Distribution calculates the probability of a specific number of successes (k) in a fixed number of trials (n), where each trial has only two possible outcomes.

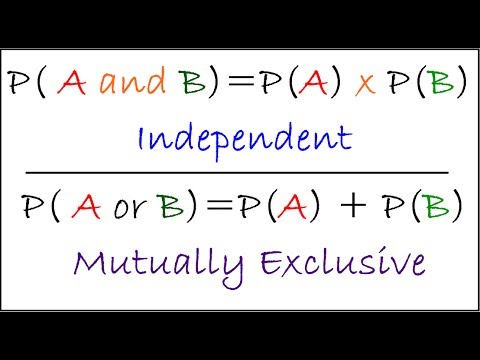
In summary, the Binomial Probability Distribution is a probability distribution used when there are only two possible outcomes, and each trial is independent of the previous one. It helps in calculating the probability of success in a fixed set of parameters.

Understanding the Target of Binomial Probability Distribution

The target of the Binomial Probability Distribution is to calculate the probability of a specific number of successes in a fixed number of independent trials that have only two possible outcomes. In simpler terms, it helps us to understand the likelihood of an event happening when there are only two possible outcomes.

For example, a coin toss has only two possible outcomes: heads or tails. The Binomial Probability Distribution can calculate the probability of getting three heads in five tosses of a coin.

Personal Experience with Binomial Probability Distribution and Its Importance

As a data analyst, I had to work on several models to predict user behavior on an e-commerce website. We used Binomial Probability Distribution to predict the chance of a user making a purchase or not. It helped us to make data-driven decisions to increase the conversion rate, which eventually helped in generating more revenue.

Using Binomial Probability Distribution, we calculated the probability of a user making a purchase based on the number of times they visited our website. It gave us a deeper insight into user behavior and helped us to take necessary measures to increase the conversion rate.

Calculating the Binomial Probability Distribution

To calculate the Binomial Probability Distribution, we need to know the probability of success (p), the number of independent trials (n), and the number of successes (k) required in the n trials. The formula for calculating the Binomial Probability Distribution is:

P(k) = (n choose k) p^k (1-p)^(n-k)

Where:

• P(k) is the probability of k successes in n trials
• n is the total number of trials
• k is the number of successful events
• (n choose k) is the Binomial Coefficient
• p is the probability of success of an individual trial

Properties of Binomial Probability Distribution

The following are some of the essential properties of Binomial Probability Distribution:

• The probability of success (p) must remain constant throughout all the trials
• The outcome of one trial should not affect the outcome of any other trial
• There can only be two possible outcomes of each trial, success or failure
• There must be a fixed number of trials (n)
• The trials must be independent of each other

Applications of Binomial Probability Distribution

The Binomial Probability Distribution has several real-life applications, some of which are:

• Predicting the probability of a person surviving a plane crash.
• Predicting the probability of a user making a purchase on an e-commerce website
• Predicting the probability of a student getting an admission offer from a university

Question and Answer

Q: What are the parameters required to calculate the Binomial Probability Distribution?

A: The parameters required are the probability of success (p), the number of independent trials (n), and the number of successes (k) required in the n trials.

Q: What is the formula for calculating the Binomial Probability Distribution?

A: P(k) = (n choose k) p^k (1-p)^(n-k)

Q: Can Binomial Probability Distribution be used for more than two outcomes?

A: No, the Binomial Probability Distribution only applies to situations where there are only two possible outcomes for each trial.

Q: What are some of the essential properties of Binomial Probability Distribution?

A: Some of the essential properties of Binomial Probability Distribution are that the probability of success (p) must stay constant throughout all the trials, the outcome of one trial should not impact the outcome of any other trial, there can only be two possible outcomes of each trial – success or failure, and there should be a fixed number of trials (n).

Conclusion of Define Binomial Probability Distribution

The Binomial Probability Distribution is a vital tool in statistics that helps us to calculate the probability of a specific number of successes in a fixed number of trials where there are only two possible outcomes. It is widely used in various fields, including finance, marketing, and education. Understanding the Binomial Probability Distribution helps in making data-driven decisions and can lead to more effective strategies for achieving higher success rates.

Gallery

PPT - The Binomial Distribution PowerPoint Presentation, Free Download

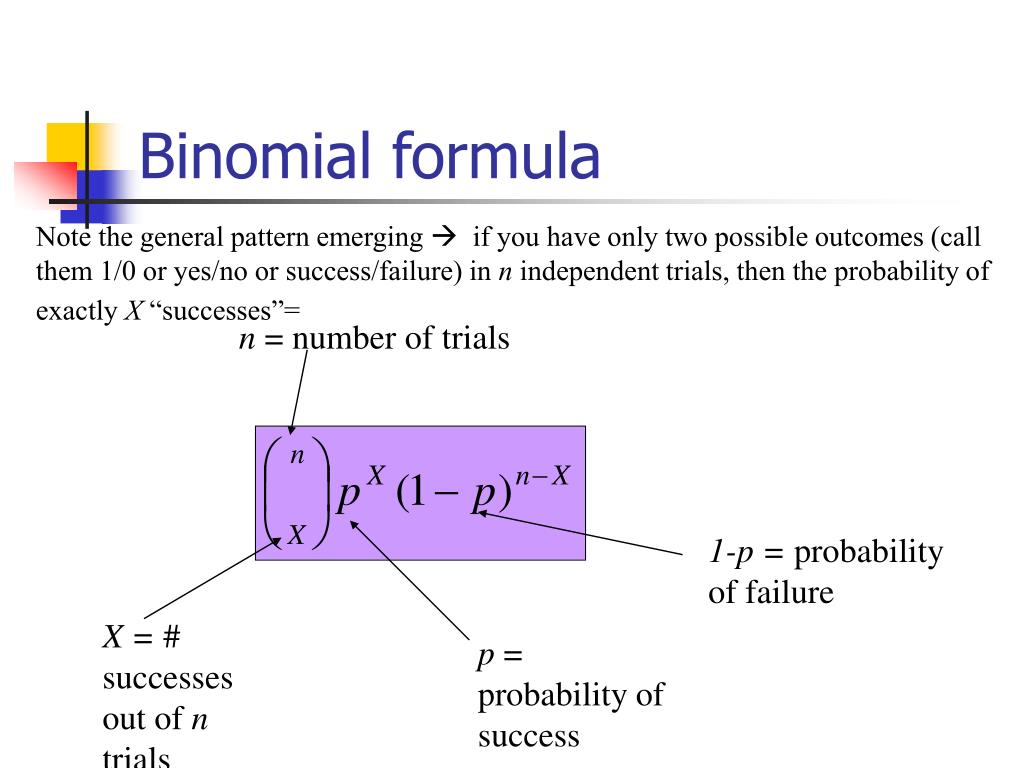
_{^{Photo Credit by: bing.com / binomial distribution formula ppt trials probability general powerpoint presentation number}}

PPT - Examples Of Discrete Probability Distributions: PowerPoint

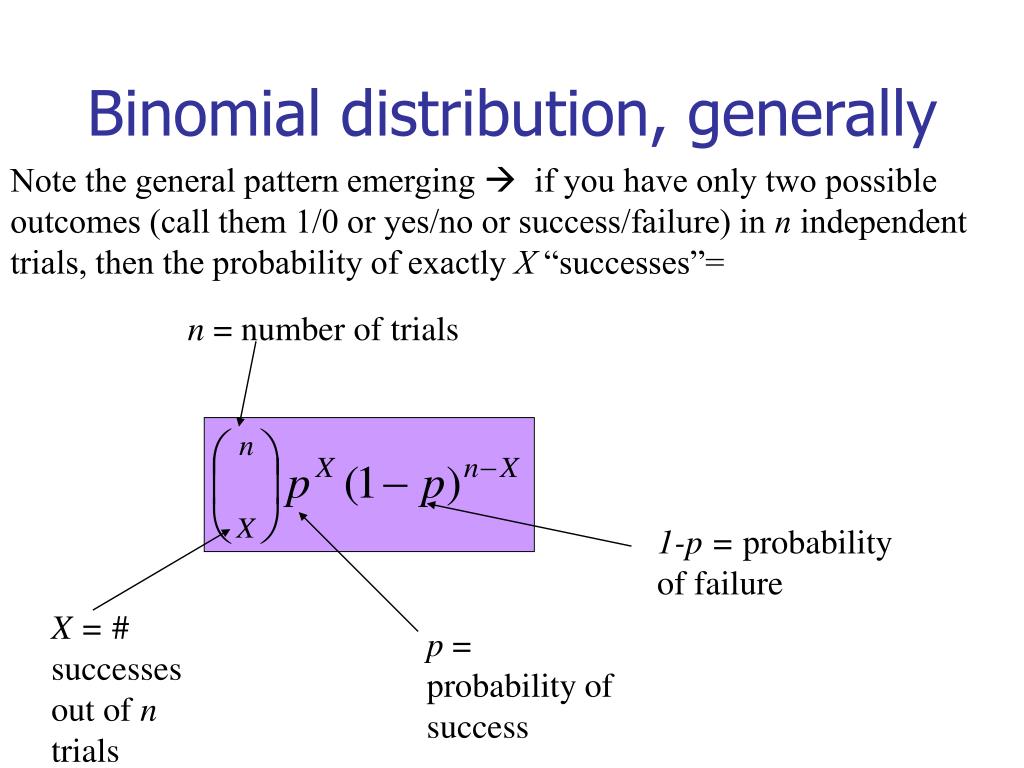
_{^{Photo Credit by: bing.com / binomial probability examples distribution discrete distributions number ppt powerpoint presentation definitions slideserve}}

PPT - Examples Of Discrete Probability Distributions: PowerPoint

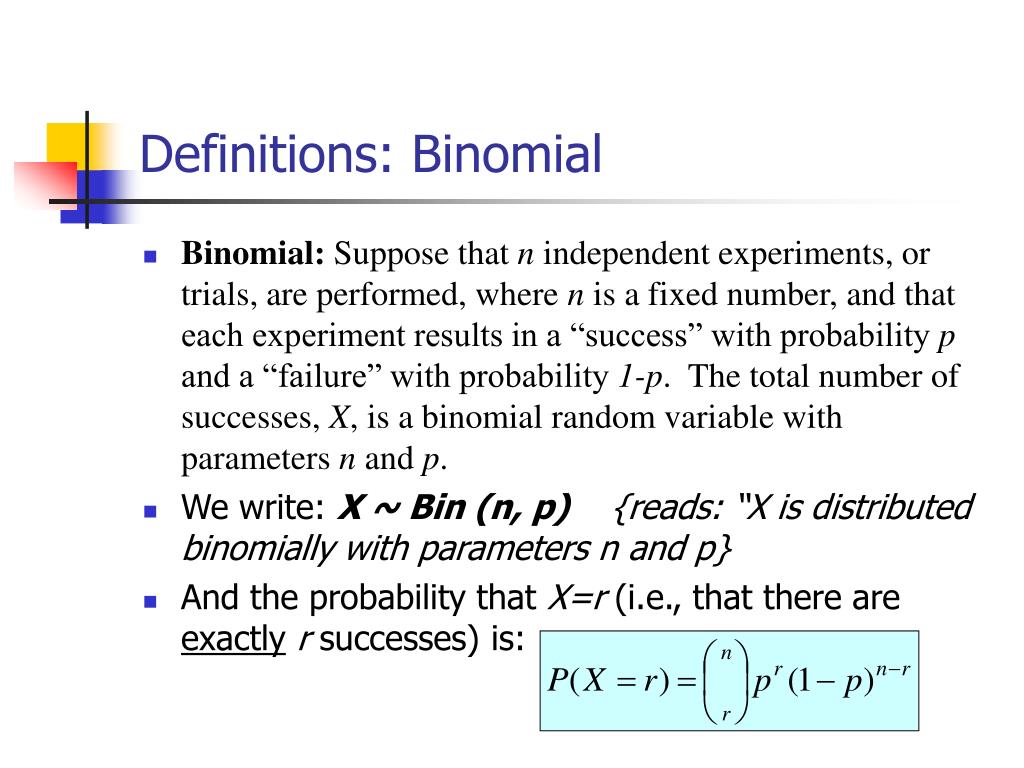
_{^{Photo Credit by: bing.com / binomial examples probability discrete distribution distributions example definitions ppt powerpoint presentation if independent slideserve}}

PPT - Examples Of Discrete Probability Distributions: PowerPoint

_{^{Photo Credit by: bing.com / binomial distribution probability examples distributions discrete example ppt getting powerpoint presentation fewer toss coin heads times if slideserve}}

Probability By Binomial Distribution: Solved Numerical Problems

_{^{Photo Credit by: bing.com / probability binomial statistics interview solved mathematics}}