Conditional Probability Formula For Independent Events

Conditional probability

Table of Contents
Understanding the Conditional Probability Formula For Independent Events
Real-World Applications of the Conditional Probability Formula For Independent Events
Putting it All Together
Question and Answer
Conclusion of Conditional Probability Formula For Independent Events
Gallery

Have you ever wondered what the chances are of two independent events happening at the same time? Or maybe you’re trying to figure out the probability of an event given that another event has already occurred? That’s where the Conditional Probability Formula For Independent Events comes in. In this post, we’ll explore what this formula is, why it’s important, and how it works.

When it comes to calculating probabilities, there are many factors to consider. The Conditional Probability Formula For Independent Events specifically deals with events that are not influenced by one another. However, it can still be a complex and confusing concept for many people to grasp.

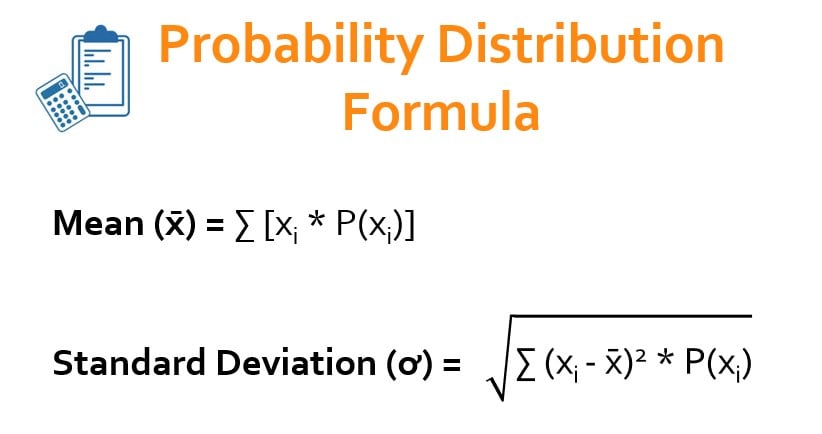
The main target of the Conditional Probability Formula For Independent Events is to calculate the probability of an event given that another event has already occurred. This formula is particularly useful in situations where multiple events need to occur in order for a particular outcome to happen.

In summary, the Conditional Probability Formula For Independent Events allows us to calculate the probability of an event given that another event has already occurred. This formula is important for understanding the chances of multiple independent events happening at the same time.

Understanding the Conditional Probability Formula For Independent Events

When working with the Conditional Probability Formula For Independent Events, it’s important to remember that the first event has no effect on the probability of the second event occurring. To calculate the probability of both events happening, you simply multiply the probability of each individual event together.

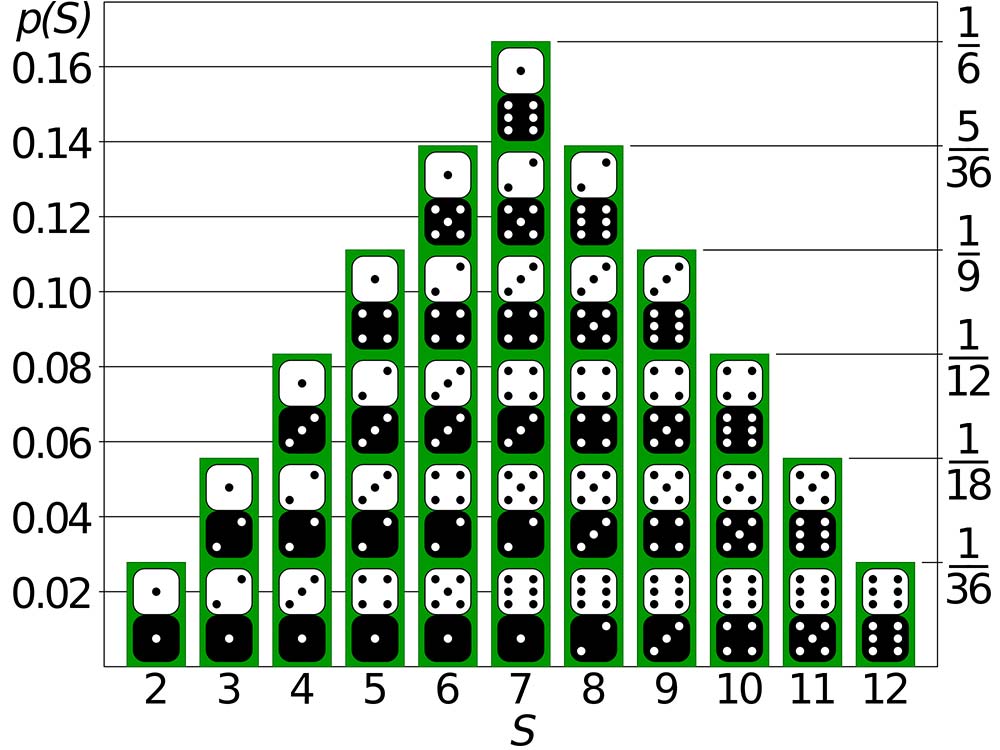
For example, let’s say that you want to figure out the probability of flipping two coins and having both of them come up heads. Since each coin flip is an independent event, the probability of both coins coming up heads is simply 1/2 x 1/2 = 1/4.

Real-World Applications of the Conditional Probability Formula For Independent Events

The Conditional Probability Formula For Independent Events can be applied to a wide variety of real-world situations. One such example is in the field of genetics. When trying to determine the probability of a certain trait being passed down from one generation to the next, the Conditional Probability Formula For Independent Events can be used.

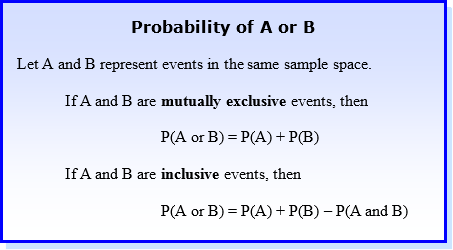Another example where this formula can be applied is in weather forecasting. Meteorologists often rely on the probability of multiple independent events happening (such as temperature, humidity, and wind direction) in order to predict the likelihood of a particular weather pattern occurring.

Calculating Conditional Probabilities for Dependent Events

While the Conditional Probability Formula For Independent Events deals specifically with events that are not influenced by one another, it’s important to note that there is also a formula for calculating conditional probabilities for dependent events. When dealing with dependent events, the probability of the second event occurring is influenced by the outcome of the first event.

The Formula for Conditional Probabilities for Dependent Events

When calculating probabilities for dependent events, the formula is slightly different. Here’s how it works:

Conditional Probability = (Probability of the First Event) x (Probability of the Second Event given that the First Event has occurred)

Putting it All Together

In conclusion, understanding the Conditional Probability Formula For Independent Events is an important part of calculating probabilities in a wide range of fields. By knowing how to calculate the probability of one event given that another event has already occurred, it becomes possible to predict the likelihood of multiple independent events happening at the same time.

Question and Answer

1. What is the Conditional Probability Formula For Independent Events?

The Conditional Probability Formula For Independent Events is used to calculate the probability of an event given that another event has already occurred.

2. What types of events does this formula deal with?

The Conditional Probability Formula For Independent Events deals specifically with events that are not influenced by one another.

3. How is the Conditional Probability Formula For Independent Events used in genetics?

The Conditional Probability Formula For Independent Events can be used to determine the probability of a certain trait being passed down from one generation to the next.

4. What is the formula for calculating conditional probabilities for dependent events?

The formula for calculating conditional probabilities for dependent events is: Conditional Probability = (Probability of the First Event) x (Probability of the Second Event given that the First Event has occurred)

Conclusion of Conditional Probability Formula For Independent Events

The Conditional Probability Formula For Independent Events is a powerful tool for calculating the probability of multiple events occurring at the same time. By understanding how this formula works and how it can be applied in real-world situations, it becomes possible to make more accurate predictions and better understand the chances of specific outcomes happening.

Gallery