solved conditional probability exercise
Table of Contents
Table of Contents
Conditional probability can be a daunting topic for many people, but it doesn’t have to be. In this blog post, we will break down Conditional Probability For Dummies, explaining the topic in straightforward terms that anyone can understand.
What Are the Pain Points Related to Conditional Probability?
Probability can be challenging to grasp, and conditional probability adds another level of complexity to the mix. It can be challenging to understand how to calculate the probability of an event occurring when we know that another event has already occurred. Many people struggle with understanding the basics and often avoid the topic altogether.
What Is the Target of Conditional Probability For Dummies?
The target of Conditional Probability for Dummies is anyone who wants to learn or improve their understanding of probability and conditional probability. From students to professionals to hobbyists, anyone can benefit from a clear understanding of this topic.
Summary of Main Points Related to Conditional Probability For Dummies
In this blog post, we will demystify the topic of conditional probability by explaining what it is and how to calculate it. We will also cover the differences between conditional and independent events, how to use Bayes’ theorem, and practical applications of conditional probability in real life. By the end of this post, you will have a solid understanding of how conditional probability works and how to apply it in different scenarios.
What Is Conditional Probability For Dummies, and Why Is It Important?
Conditional Probability For Dummies is an entry-level guide to understanding probability and conditional probability. It explains concepts such as independence, dependence, and the relationship between events. For the uninitiated, it’s an essential tool in understanding how to calculate probability when events are dependent on each other.
My first experience with conditional probability was in high school math class. I struggled to understand the concept and found myself completely lost. It wasn’t until I read an entry-level guide that I finally grasped the basics and was able to build upon that foundation.
Conditional Probability For Dummies is an essential tool for anyone looking to improve their foundational understanding of probability and conditional probability. It provides readers with the necessary tools to calculate probabilities in real-life scenarios with ease.
Why Is Understanding Conditional Probability Important?
Conditional probability is essential in various fields, from data science and finance to medicine and sports analytics. Probability allows for better decision-making, as it provides us with the tools to predict outcomes accurately. Understanding conditional probability is especially crucial for anyone involved in making data-driven decisions.
For example, let’s say a doctor wants to determine the probability of a disease in a patient’s test result, given that they are exhibiting specific symptoms. By understanding conditional probability, the doctor can make an accurate diagnosis and recommend appropriate treatment.
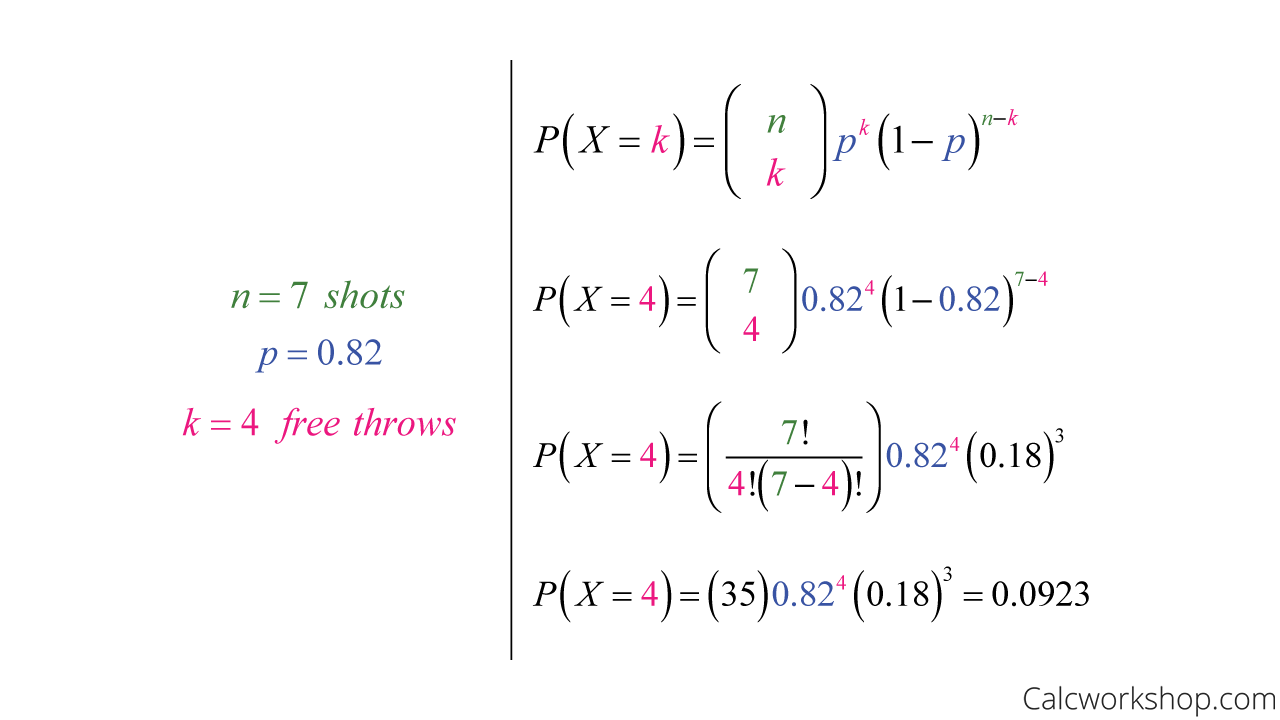
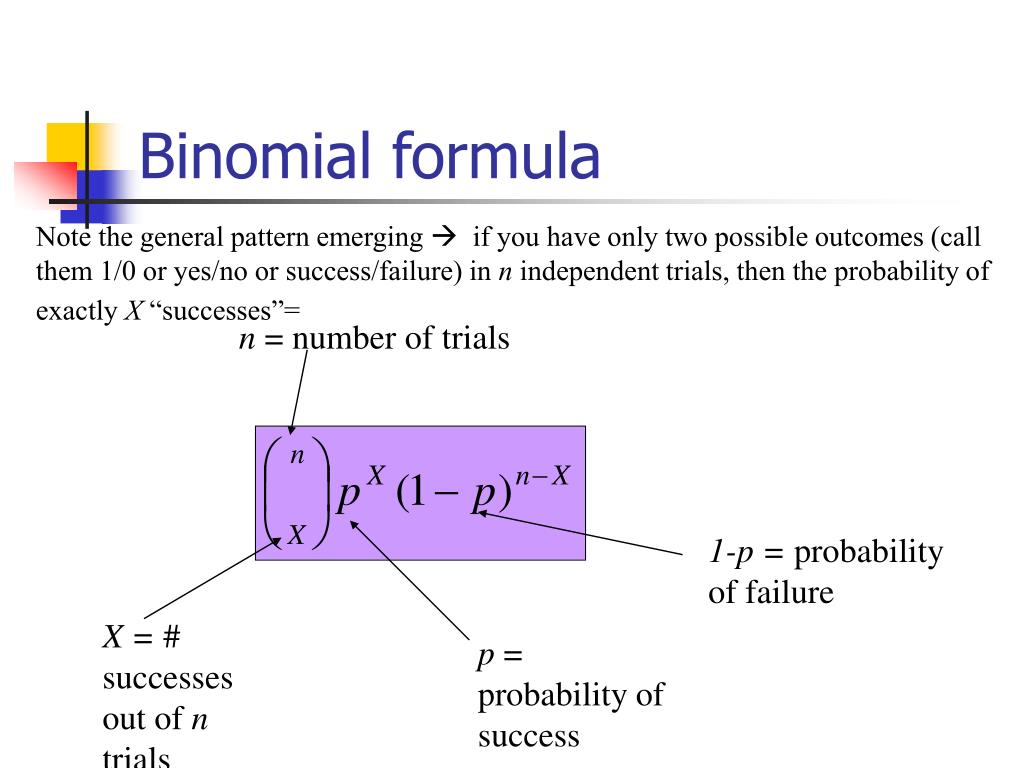
How to Calculate Conditional Probability?
The formula for conditional probability is straightforward:
P(A|B) = P(A and B) / P(B)
This formula tells us the probability of event A occurring, given that event B has already occurred.
For example, let’s say we have a jar of marbles containing five red and five green marbles. If we want to know the probability of drawing a red marble, given that we have already drawn a green marble, the formula would look like this:
P(Red|Green) = P(Red and Green) / P(Green)
Since we have already drawn a green marble, there are now four green and five red marbles remaining. Therefore:
P(Red|Green) = (5/10 x 4/9) / (5/10) = 2/9
Bayes’ Theorem
Bayes’ theorem is another essential tool in calculating conditional probability. It allows us to calculate the probability of an event based on prior knowledge.
Bayes’ theorem can be expressed in the following formula:
P(A|B) = P(B|A) P(A) / P(B)
This formula tells us the probability of event A given the occurrence of event B and our prior knowledge of P(A) and P(B).
Real-Life Applications of Conditional Probability
Conditional probability has numerous real-life applications. In finance, it can be used to predict stock prices and make trading decisions. In sports analytics, it can be used to predict the outcomes of games, and in medicine, it can be used to make accurate diagnoses.
For example, consider the case of a casino. Casinos use probability to their advantage, hedging their bets on the likelihood of an event occurring. By analyzing probabilities of a game, a casino can make decisions on which games to offer or which ones to avoid, maximizing their profits.
Question and Answer
Q. What is the difference between independent and dependent events?
A. Independent events are events where the outcome of one event does not affect the outcome of the other event. In contrast, dependent events are events where the outcome of one event affects the outcome of the other event.
Q. How can Bayes’ theorem be used in real life?
A. Bayes’ theorem can be used in real life in various fields such as medicine, finance, and marketing. For example, Bayes’ theorem can be used in medicine to determine the probability of a patient having a disease given their symptoms and medical history.
Q. How does conditional probability differ from joint probability?
A. Conditional probability is the probability of an event occurring, given that another event has occurred. Joint probability, on the other hand, is the probability of two events occurring simultaneously.
Q. What is the formula for conditional probability?
A. The formula for conditional probability is P(A|B) = P(A and B) / P(B).
Conclusion of Conditional Probability For Dummies
Conditional Probability For Dummies may seem like a daunting topic, but it doesn’t have to be. In this blog post, we covered the basics of probability and conditional probability, including the differences between independent and dependent events, the formula for conditional probability, and the real-life applications of this important tool. By understanding conditional probability, anyone can make more accurate predictions and better-informed decisions in their personal and professional lives.
Gallery
Conditional Probability Digital Task Cards - Lindsay Bowden

Photo Credit by: bing.com / probability conditional task cards digital cart
Conditional Probability

Photo Credit by: bing.com /
PPT - 5.3 Conditional Probability PowerPoint Presentation, Free
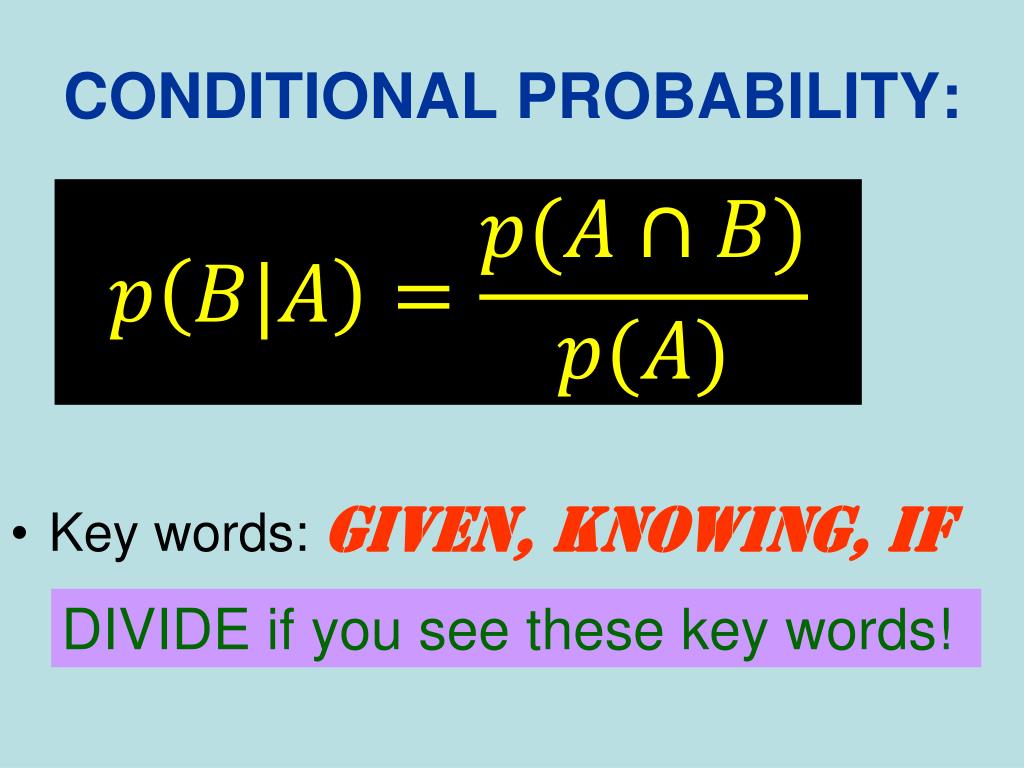
Photo Credit by: bing.com /
Conditional Probability

Photo Credit by: bing.com /
[Solved] Conditional Probability Exercise | 9to5Science
![]()
Photo Credit by: bing.com /